Asymmetric Propeller of Squares II
What Is This About?
Problem
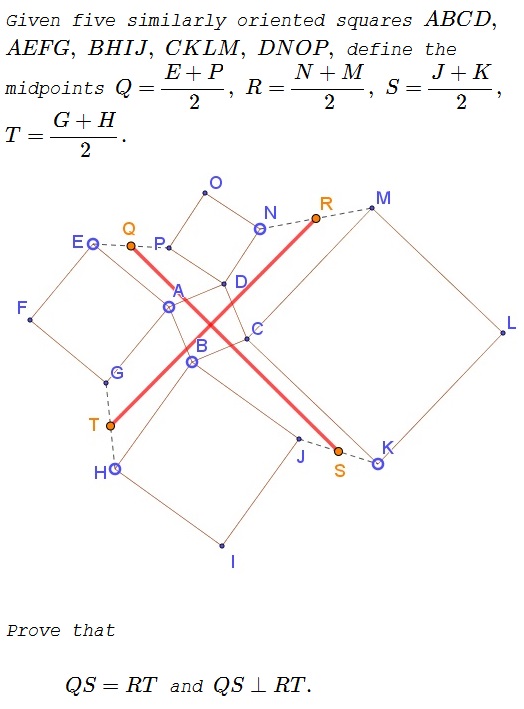
Solution
We'll use the lower case letter corresponding to the names of of the points as associated complex numbers. We also assume, $a=1,$ $b=i,$ $c=-1,$ $d=-i.$ Then, for example, $(g-a)=i(e-a)$ so that $g=a+i(e-a).$ Similarly,
$\displaystyle\begin{align} j&=b+i(h-b),\\ m&=c+i(k-c),\\ p&=d+i(n-d). \end{align}$
Further,
$\displaystyle \begin{align} Q&=\frac{E+P}{2}=\frac{e+p}{2}=\frac{e+d+i(n-d)}{2},\\ R&=\frac{N+M}{2}=\frac{n+m}{2}=\frac{n+c+i(k-c)}{2},\\ S&=\frac{J+K}{2}=\frac{j+k}{2}=\frac{k+b+i(h-b)}{2},\\ T&=\frac{H+G}{2}=\frac{h+g}{2}=\frac{h+a+i(e-a)}{2}. \end{align}$
Further,
$\displaystyle \begin{align} 2(q-s)&=[e+d+i(n-d)]-[k+b+i(h-b)]\\ &=[e+d-k-b]+i[n-d-h+b]\\ &=[e-k]+i[n-h]+2(i-1),\\ 2(r-t)&=[n+c+i(k-c)]-[h+a+i(e-a)]\\ &=[n+c-h-a]+i[k-c-e+a]\\ &=[n-h]+i[k-e]+2(i-1)\\ &=-i\{[e-k]+i[n-h]\}+2(i-1), \end{align}$
because $(d-b)(1-i)=2(i-1)=(c-a)(i-1).$ It follows that $r-t=i(s-q).$
Acknowledgment
This problem grew out of another one and was proposed by Alexandr Skutin.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73299329
