Parallelogram in Trapezoid
Source
Leo Giugiuc has kindly posted a problem by Miguel Ochoa Sanchez at the CutTheKnotMath facebook page along with his and Dan Sitaru's solution (Proof 1).
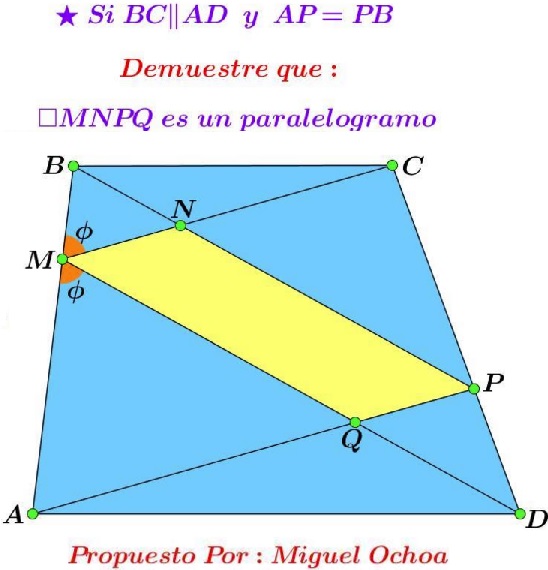
Problem
In trapezoid $ABCD,\;$ $BC\parallel AD,\;$ $P\in CD\;$ satisfies $AP=BP;\;$ $M\in AB,\;$ with $\angle AMD=\angle BMC;\;$ $N=BP\cap CM\;$ and $Q=AP\cap DM.$
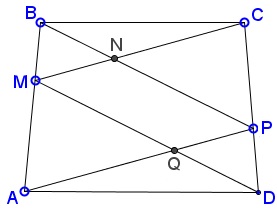
Prove that the quadrilateral $MNPQ\;$ is a parallelogram.
Proof 1
Choose $A=(1,0),\;$ $B=(-1,0),\;$ and $P=(0,a),\;$ with $a\gt 0.\;$ Since $P\in CD,\;$ and $CD\;$ does not cross the interior of $\Delta APB,\;$ there is $m\in (-a,a)\;$ such that $CD\;$ is defined by the equation $-mx+y=a.\;$ Also, since $BC\parallel AD,\;$ and neither passes through the interior of $\Delta APB,\;$ there is $\displaystyle n\in\left(-\frac{1}{a},\frac{1}{a}\right)\;$ such that $BC\;$ and $AD\;$ are defined by $x-ny=-1\;$ and $x-ny=1,\;$ respectively.
These give us $\displaystyle C=\left(\frac{na-1}{1-mn},\frac{a-m}{1-mn}\right)\;$ and $\displaystyle D=\left(\frac{na+1}{1-mn},\frac{a+m}{1-mn}\right).\;$ Assume $M=(k,0).\;$ Since $\angle BMC=\angle AMD,\;$ the lines $MC\;$ and $MD\;$ have opposite antislopes. Thus
$\displaystyle\frac{k-\displaystyle\frac{na-1}{1-mn}}{\displaystyle\frac{a-m}{1-nm}}=\frac{-k+\displaystyle\frac{na+1}{1-mn}}{\displaystyle\frac{a+m}{1-nm}},$
implying $\displaystyle k=\frac{na^2-m}{a(1-mn)}.\;$ Using this, we can easily check that $MC\parallel AP\;$ and $MD\parallel BP.\;$ Thus $MNPQ\;$ is indeed a parallelogram.
Proof 2
Find point $M'\;$ on $AB\;$ such that $\angle BM'C=90^{\circ}-\frac{1}{2}\angle APB.$ From $M'\;$ draw a ray $M'D',\;$ with $D'\;$ on line $CP\;$ such that $\angle CM'D'=\angle APB.\;$ Then, as we know, $AD'\parallel BC,\;$ so, since also $AD\parallel BC,\;$ we see that $D'=D.\;$ Thus $M'\;$ solves Heron's problem for $C\;$ and $D\;$ and, as such, is unique on $AB\;$ with the property that $\angle BM'C=\angle AM'D.\;$ It follows that $M=M'.$
From the construction of $M',\;$ the quadrilateral $MNPQ\;$ is a parallelogram.
Proof 3
This proof is be Grégoire Nicollier.
Angles $BPA\;$ and $CMD\;$ have parallel bisectors. Suppose that angle $CMD\;$ is larger. $PB\;$ and $DM\;$ would intersect at some $I\;$ left from $AB\;$ and above $BC;\;$ $PA\;$ and $CM\;$ would intersect at a $J\;$ left from $AB\;$ and below $AD.\;$ $IJ\;$ would intersect $BC.\;$ Analogous when angle $CMD\;$ is smaller than $BPA.\;$
This would contradict Pappus' theorem! In hexagon $BPADMC,\;$ whose vertices are alternately on two lines, there is one pair $BC,\;AD\;$ of parallel opposite sides. If not parallel, the other opposite sides would not intersect in two points that lie on a line parallel to $BC,\;$ a contradiction with Pappus.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73300295
