From Straight Line to Regular Hexagon
What Might This Be About?
Problem
Let $BED,HIF, DLJ,FGA, ACB,JKH$ be six equilateral triangles such that $\Delta BED=\Delta HIF,$ $\Delta DLJ=\Delta FGA,$ $\Delta ACB=\Delta JKH$ and $AF+BD=AB.$
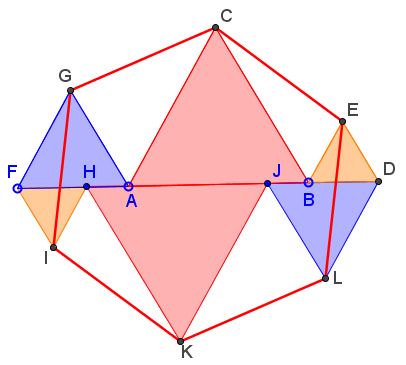
Then $GCELKI$ is a regular hexagon and $\displaystyle [GCELKI]=\frac{3\sqrt{3}}{4}(AF^2+AB^2+BD^2),$ where $[X]$ denotes the area of figure $X.$
Solution
The proof proceeds in three steps:
First we prove that $GCELKI$ is equilateral. Then we find that all its vertices are equidistant from the midpoint of $M$ of $DF.$ The required area formula is established on the third step as a consequence of the formulas obtained on the first two.
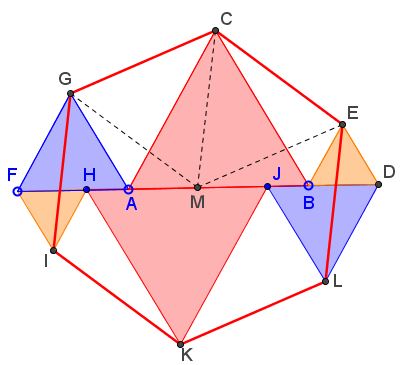
The main tool is the Law of Cosines. Denote $AF=a,$ $BD=b,$ $AB=c=a+b.$
Thus, in $\Delta BCE,$ $CE^{2}=c^{2}+b^{2}-bc;$ in $\Delta ACG,$ $CG^{2}=c^{2}+a^{2}-ac;$ in $\Delta FGI,$ $GI^{2}=a^{2}+b^{2}+ab.$
Now observe that all three expressions give the same result:
$\begin{align} c^{2}+b^{2}-bc &= c^{2}-b(c-b)=c^{2}-ab,\\ c^{2}+a^{2}-ac &= c^{2}-a(c-a)=c^{2}-ab,\\ a^{2}+b^{2}+ab &= a^{2}+b^{2}+2ab - ab = (a+b)^{2}-ab=c^{2}-ab. \end{align}$
Now introduce Cartesian coordinates with $F=(0,0).$ Then $M=(a+b,0),$ $\displaystyle G=\bigg(\frac{a}{2},\frac{\sqrt{3}a}{2}\bigg),$ $\displaystyle C=\bigg(\frac{3a+b}{2},\frac{\sqrt{3}(a+b)}{2}\bigg),$ $\displaystyle B=\bigg(2a+\frac{3b}{2},\frac{\sqrt{3}b}{2}\bigg).$ If $d(P,Q)$ stands for the square of the distance between points $P$ and $Q$ then
$\begin{align}\displaystyle d(G,M)&=\bigg(\frac{a}{2}+b\bigg)^{2}+\bigg(\frac{\sqrt{3}a}{2}\bigg)^{2}\\ &=a^{2}+b^{2}+ab. \end{align}$
Similarly,
$\begin{align}\displaystyle d(C,M)&=\bigg(\frac{a}{2}-\frac{b}{2}\bigg)^{2}+\bigg(\frac{\sqrt{3}(a+b)}{2}\bigg)^{2}\\ &=a^{2}+b^{2}+ab. \end{align}$
Finally,
$\begin{align}\displaystyle d(B,M)&=\bigg(a+\frac{b}{2}\bigg)^{2}+\bigg(\frac{\sqrt{3}b}{2}\bigg)^{2}\\ &=a^{2}+b^{2}+ab. \end{align}$
For the area formula, by the sine formula for the area of a triangle,
$\begin{align}\displaystyle [GCELKI]&=6[\Delta CMG]\\ &=6\cdot\frac{1}{2}\cdot\frac{\sqrt{3}}{2}(a^{2}+b^{2}+ab)\\ &=\frac{3\sqrt{3}}{4}(a^{2}+b^{2}+(a+b)^{2}). \end{align}$
Acknowledgment
The construction has been posted by Oai Thanh Dào at the CutTheKnotMath facebook page. This is a special case of a more general result.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73293904
