Equal Areas in Circle
Source
Leo Giugiuc has kindly posted at the CutTheKnotMath facebook page another problem by Rubén Dario Auqui and a solution by Claudia Nanuti, Diana Trailescu, Dan Sitaru and Leo Giugiuc:
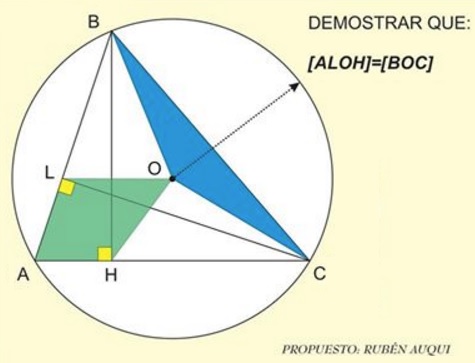
Problem
In $\Delta ABC,$ $O$ is the circumcenter; $BH$ and $CL$ are two altitudes:

Prove that the areas $[ALOH]$ and $[BOC]$ are equal.
Solution
Let $M,$ $N,$ $P$ be the midpoints of the sides $BC,$ $AC,$ and $AB,$ respectively; $\alpha =\angle BAC = \angle BOM;$ $R=OB,$ the circumradius of $\Delta ABC.$ Then $OM=R\cdot\cos\alpha.$ If $\beta =\angle ABC$ and $\gamma =\angle ACB$ then, similarly, $ON=R\cdot\cos\beta$ and $OP=R\cdot\cos\gamma.$
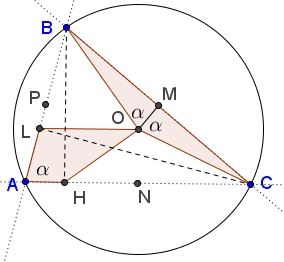
In addition, by the Law of Sines,
$AH=AB\cdot\cos\alpha =2R\cdot\sin\gamma\cdot\cos\alpha,\\ AL=AC\cdot\cos\alpha=2R\cdot\sin\beta\cdot\cos\alpha.$
Observe that
$\begin{align} [ALOH] &= [\Delta ALO]+[\Delta AHO]\\ &=\frac{1}{2}AL\cdot OP+\frac{1}{2}AH\cdot ON\\ &=R^2\cos\alpha\cdot (\sin\beta\cdot\cos\gamma+\sin\gamma\cdot\cos\beta)\\ &=R^2\cos\alpha\cdot\sin (\beta+\gamma )\\ &=R^2\cos\alpha\cdot\sin\alpha\\ &=\frac{1}{2}R^2\sin 2\alpha\\ &=[BOC]. \end{align}$
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73293936
