Dao's Six Point Circle

What Might This Be About?

Problem

The centers of the six circles tangent to the medians of $\Delta ABC$ at the centroid and through the vertices of the triangle are concyclic:

Circles Tangent to Medians at the Centroid - problem

Solution

Let $A', B', C'$ be the midpoints of the sides of $\Delta ABC;$ $A'',$ $B'',$ $C''$ the midpoints of $AG, BG, CG$, respectively, and let the perpendicular bisectors of $AG, BG, CG$ meet at $K, L, M;$ finally, let $A_{B},$ $A_{C},$ $B_{C},$ $B_{A},$ $C_{A},$ $C_{B}$ be the centers of the mentioned six circles.

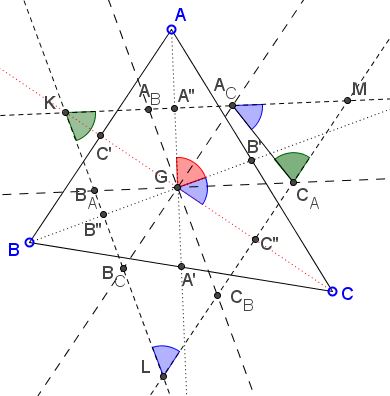
Circles Tangent to Medians at the Centroid - solution

Since $GA''\perp KM$ and $GC''\perp LM,$ quadrilateral $A''GC''M$ is cyclic, implying

(1)

$\angle A_{C}C_{A}M + \angle C_{A}A_{C}M = \angle AGB' + \angle B'GC.$

As $B'$ is the midpoint of $AC$, we have

$\displaystyle \frac{\sin\angle AGB'}{\sin\angle B'GC} = \frac{GC}{GA} =\frac{GC''}{GA''}=\frac{GC_{A}}{GA_{C}} $

because $\Delta GA''A_{C}\sim \Delta GC''C_{A}$. And, to contninue,

$\displaystyle\frac{GC_{A}}{GA_{C}}=\frac{MA_{C}}{MC_{A}} = \frac{\sin\angle C_{A}A_{C}M}{\sin\angle A_{C}C_{A}M},$

so that

(2)

$\displaystyle\frac{\sin\angle AGB'}{\sin\angle B'GC} = \frac{\sin\angle A_{C}C_{A}M}{\sin\angle C_{A}A_{C}M}.$

(1) and (2) give $\angle AGB' = \angle A_{C}C_{A}M$ as well as $\angle B'GC = \angle C_{A}A_{C}M.$ Further, $\angle AGB' = \angle MKL$ because the sides are pairwise perpendicular. Hence, $A_{C}C_{A}$ is an antiparallel in $\Delta KLM.$ By analogy, so are $A_{B}B_{A}$ and $B_{C}C_{B},$ showing that the six centers form a Tucker hexagon and lie on a Tucker circle.

Acknowledgment

This problem has been posted by Dao Thanh Oai (Vietnam) at the CutTheKnotMath facebook page. The solution is a modification of the one posted at a Vietnamese math site under the moniker Vslmat.

71532905