The length of the diagonal of the unit square equals the square root of 2.

The length of the diagonal of the unit square equals 2. Well, this is not really great news. Every one who ever came across the Pythagorean theorem is aware of the fact. In the isosceles right triangle with the legs of length 1, the hypotenuse, according to the Pythagorean theorem - 1² + 1² = (hypotenuse)² - is indeed of length 2. The motivation for this page is not so much that fact itself, but the way of showing it without a recourse to the famous theorem. Indeed, it could be argued that the Pythagorean theorem might have been overused, see a curious example.

An argument that goes back to the figure drawn by Socrates in Plato's Meno might have been known to Pythagoras himself, although the latter lived a century earlier.

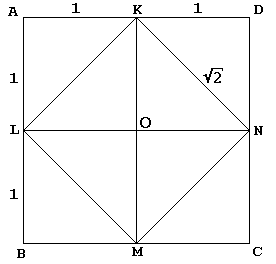
In the dialog, Socrates, in his usual manner, leads a young slave to a construction of a square twice the area of the given one:

The given square KLMN with center O is first cut by its diagonals into 4 equal triangles. Socrates embeds KLMN into a bigger square ABCD as if reflecting these triangles each in its hypotenuse. The slave then admits - and who would not? - that square ABCD is twice as big as square KLMN.

Truth be told, Socrates' square ABCD measured 4 on the side, such that square KLMN had area of 8. It is clear that when we start with the square ABCD of side 2 (and hence of area 4), square KLMN will have area of 2.

Now, in general, for a positive number A, A is defined as a number whose square is A:

(A)2.

On the other hand, every student of areas knows, that the area of a square of side b equals b². It follows then that the side of a square with area A is exactly A. We conclude that the side of the square KLMN equals 2. For one,

KN = A.

The central point of the argument is the fact that, in the diagram, KN plays a double role. Besides being one of the sides of square KLMN, it also serves as a diagonal of square OKDN. The latter is of course a unit square, which proves our assertion.

(From a little different perspective the same episode is mentioned elsewhere.)

References

1. B. Mazur, Imagining Numbers, Farrar Straus Giroux, 2003