Dan Sitaru's Sum of a Series
Problem
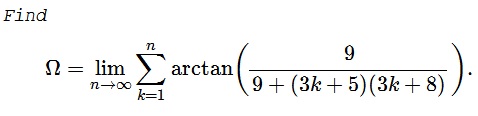
Solution 1
$\displaystyle \begin{align} \Omega &= \lim_{n\rightarrow\infty}\sum_{k=1}^{n}\arctan\left(\frac{9}{9+(3k+5)(3k+8)}\right)\\ &= \lim_{n\rightarrow\infty}\sum_{k=1}^{n}\left[\arctan\left(k+\frac{8}{3}\right)-\arctan\left(k+\frac{5}{3}\right)\right]\\ &= \lim_{n\rightarrow\infty}\left[\arctan\left(n+\frac{8}{3}\right)-\arctan\left(\frac{8}{3}\right)\right]\\ &=\frac{\pi}{2}-\arctan\frac{8}{3}=\arctan\frac{3}{8}. \end{align}$
Solution 2
We will use the property that: for $-1\lt x,y\lt 1$,
$\displaystyle \arctan(x)+\arctan(y)=\arctan\left(\frac{x+y}{1-x y}\right)$
Let $\displaystyle U(k)=\frac{9}{(3 k+5) (3 k+8)+9}$.
We need to solve the recurrent equation:
$\displaystyle f(k)=\frac{f(k-1)+U(k)}{1-f(k-1) U(k)}, \text{ w. init. cond. } f(1)=\frac{9}{97}.$
Which yields the solution $\displaystyle f(k)= \frac{9 k}{24 k+73}$.
We have
$\displaystyle \lim_{k\to \infty } \frac{9 k }{24 k+73}=\frac{3}{8}$
Hence $\displaystyle \Omega = \arctan(\frac{3}{8})$.
Solution 3
Let,
$\displaystyle \tan \theta_k = \frac{9}{9+(3k+5)(3k+8)},~\tan \alpha_k = k+\frac{5}{3}.$
Thus,
$\displaystyle \begin{align}\tan(\alpha_{k+1}-\alpha_{k})&=\frac{\tan\alpha_{k+1}-\tan\alpha_k}{1+\tan\alpha_{k}\tan\alpha_{k+1}}=\frac{1}{1+\left(k+\frac{5}{3}\right) \left(k+\frac{8}{3}\right)}\\ &= \frac{9}{9+(3k+5)(3k+8)} = \tan\theta_k. \end{align}$
Hence,
$\displaystyle \theta_k = \alpha_{k+1}-\alpha_k + M\pi, ~(\forall M \in\mathbb{Z}).$
Retaining the principal solution,
$\displaystyle \begin{align} \lim_{N->\infty} \sum_{k=1}^N \theta_k &= \lim_{N\to\infty}\sum_{k=1}^N(\alpha_{k+1}-\alpha_k) =\lim_{n->\infty} \alpha_n - \alpha_1 \\ &=\frac{\pi}{2}-\tan^{-1}\frac{8}{3} = \tan^{-1} \frac{3}{8}. \end{align}$
Acknowledgment
Dan Sitaru as kindly posted the above problem at the CutTheKnotMath facebook page, along with a solution (Solution 1) by Diego Alvariz. Serban George Florin and Youssef Khiari gave practically an identical proof. Solution 2 is by N. N. Taleb; Solution 3 is by Amit Itagi. The problem was originally published at the Romanian Mathematical Magazine.
|Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73300753
