Euler's formula for e^iθ
A geometric riff on an analytic theme

Scott E. Brodie

An intuitive understanding of Euler's formula for the complex exponential,

e^iθ = cos θ + i sin θ,

remains elusive, notwithstanding hundreds of years of contemplation. While the formula can be "motivated" or "justified" by rearrangement of the infinite series for the sine, cosine, and exponential functions, this argument requires a deeper understanding of the theory of infinite series than is likely available when Euler's formula is first encountered. A persuasive argument can also be had by demanding that differentiation of the complex exponential obey the chain rule, which leads to simple differential equations whose unique solutions recover Euler's formula [Allendoerfer], but, again, one must appeal to results from a more advanced course.

In this note, I suggest a simple heuristic motivation for Euler's formula which requires only simple geometry and limiting arguments which are within reach of a well-informed high-school student. While the argument cannot be called a "proof," for reasons which are indicated below, the construction is highly suggestive, and perhaps explains Euler's formula in a more concrete way than those based purely on analytic reasoning.

The number e is ordinarily defined by the limit

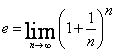
This definition for e as a limit implies a simple formula for the powers of e, which is surely valid at least for positive real exponents:

(*)

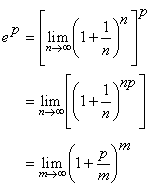
where the first step follows from the continuity of the power function, and the second step is obtained by the substitution m = np, n = m/p, or 1/n = p/m, since m = np goes to infinity whenever n does.

While, on its face, the first line in (*) makes little sense if we take p = iθ, the third line in (*) is just the limit of a sequence of complex numbers, each of which is simply a complex number raised to an integer power -- this format avoids the uninterpretable imaginary exponent.

In fact, we show below that

As an added benefit, we can apply the geometric interpretation of complex number arithmetic to visualize each term of the sequence. The magnitude of the mth term is

,

while the argument (the angle from the positive x-axis) of the m^th term is

m tan^-1 (θ / m).

The problem with this program as a "proof" of the Euler equation is the substitution step in (*). In the case of a positive real exponent p, the sequence of values of m = np approaches infinity in the same sense as the original sequence of indices n, and the usual definition of the limit of a sequence applies without difficulty. This of course is not true for a sequence of the form

m = inθ

as the definition of the limit of a sequence (for each ε > 0there exists an M such that m > M implies ...) no longer makes sense - the complex numbers are not ordered.

In order to visualize the approach to the limit

consider an explicit example, say θ = 2 (Figure 1.)

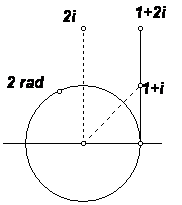	Figure 1

The first element of the sequence (1 + 2i/n)ⁿ is 1 + 2i, which corresponds to the point (1, 2). This point, and the second element of the sequence, (1 + i)² = 2i, are shown in the left-hand panel of the figure. The right hand panel illustrates the construction of the 4^th element of the sequence, (1 + i/2)⁴. The magnitude of the factor 1 + i/2 is slightly greater than 1.12; the argument is tan^-1(1/2), about 26.6° or 0.46 radians. The magnitude of the 4^th power is about 1.56; the argument of the 4^th power is about 106.3°, or approximately 1.86 radians.

A similar plot of the construction of (1 + 2i/10)¹⁰is shown in Figure 2:

	Figure 2

Evidently, as n increases, the successive points of the form (1 + 2i/n)^k more and more closely approach the unit circle. With increasing n, the argument of the nth power appears to approach 2 radians. The nature of the approach to the limit is illustrated in the Figure 3., which shows a plot of the first 30 terms of the sequence (1 + 2i/n)ⁿ. (Each dot in this figure corresponds to the "last" dot of a figure similar to the ones above):

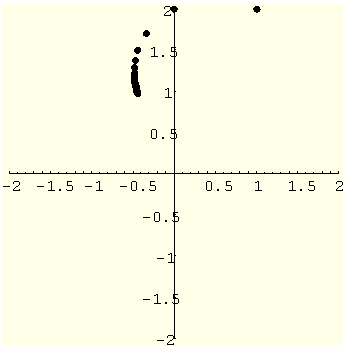	Figure 3

In other words, with increasing n, the points (1 + 2i/n)^k, (k = 1 to n), appear to ever more closely "wrap" around the unit circle, approaching as a limit the point on the circle at an angle of 2 radians from the positive real axis. But the identity of this point is well known: with Cartesian coordinates of (cos 2, sin 2), it is the complex number cos 2 + isin 2. Indeed, the approach to this limit realizes in a concrete way the "wrapping function" of elementary trigonometry!

Of course, there is nothing special about the value θ = 2. In order to prove the result in the general case, it is convenient to analyze the magnitude and the argument of the limit point separately.

First, consider the magnitude. We must show that

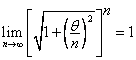
There is no point in retaining the square-root: we may as well show that

,

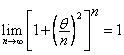since a sequence of positive real numbers and the sequence of its square roots approach 1,or not,together.

First, it let's put the exponent back on the outside of the square brackets:

Thus, we need only show that

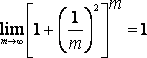
To this end, expand in terms of the binomial theorem:

Of course,

,

so taking the limit as m increases without bound, we have

,

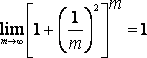QED.

(This calculation can be greatly simplified by the use of logarithms, but that seems contrary to the spirit of this exercise.)

Now consider the argument of the limit point. We must show that

The geometry is shown in the figure:

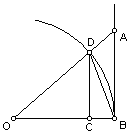
O is the origin; B is the point (1,0), and A is the point

1 + iθ / n

Denote the angle DOB by θ_n. Then tan θ_n = θ/n, or θ_n = tan^-1 (θ/n). Now compare the areas of triangle DOB = ½ sin θ_n , sector DOB = ½ θ_n , and triangle AOB = ½ tan θ_n. By inclusion, after canceling a common factor of ½, we have

sin θ_n< θ_n< tan θ_n.

Now sin θ_n= CD. Since triangle DCO is similar to triangle ABO, we have

.

Thus

,

since tan θ_n = θ/n.

Now multiply through by n:

.

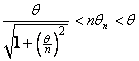Now take the limit as n increases without bound:

,

or

,

QED.

Reference

1. Allendoerfer CB, Editorial - The Proof of Euler's Equation. American Mathematical Monthly 55(2) (Feb, 1948), pp 94-95.

Appendix

One can sidestep a close analysis in terms of the binomial coefficients by taking logarithms. To avoid stray coefficients one works with natural logarithms (that is, logarithms with base e), for which log(1 + u) ~ u for small values of u. Then

Taking the limit as n grows without bound, we have

,

or

,

since the logarithm is continuous.

|Contact| |Front page| |Contents| |Geometry|

Copyright © 1996-2018 Alexander Bogomolny