Determinant on a Circle

Leo Giugiuc has kindly posted on the CutTheKnotMath facebook page a problem due to Vasile Masgras, with a solution of his own:

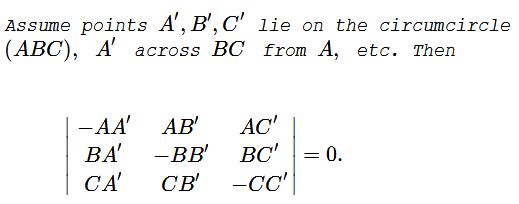
Determinant on a Cicle

Solution

|Contact| |Front page| |Contents| |Algebra|

Copyright © 1996-2018 Alexander Bogomolny

Assume points $A',B',C'$ lie on the circumcircle $(ABC),\;$ $A'\;$ across $BC\;$ from $A,\;$ etc. Then

$\begin{vmatrix} -AA'&AB'&AC'\\BA'&-BB'&BC'\\CA'&CB'&-CC' \end{vmatrix}=0.$

Solution

Observe that

$abc\begin{vmatrix} -AA'&AB'&AC'\\BA'&-BB'&BC'\\CA'&CB'&-CC' \end{vmatrix}=\begin{vmatrix} -aAA'&aAB'&aAC'\\bBA'&-bBB'&bBC'\\cCA'&cCB'&-cCC' \end{vmatrix},$

where, as usual, $a,b,c\;$ are the side length of $BC,CA,AB,\;$ respectively. By Ptolemy's theorem, $aAA'=bA'+cCC',\;$ $bBB'=aAB'+cCB',\;$ $cCC'=aAC'+bBC'.\;$ Thus adding the last two rows to the first we get a row of zeros which shows that the whole determinant is $0:$

$abc\begin{vmatrix} -AA'&AB'&AC'\\BA'&-BB'&BC'\\CA'&CB'&-CC' \end{vmatrix}=\begin{vmatrix} -aAA'&aAB'&aAC'\\bBA'&-bBB'&bBC'\\cCA'&cCB'&-cCC' \end{vmatrix}=0.$

Hence, also $\begin{vmatrix}-AA'&AB'&AC'\\BA'&-BB'&BC'\\CA'&CB'&-CC'\end{vmatrix}=0.$

Related material
Read more...

  • What Is Determinant?
  • Determinant and Divisibility
  • Determinants, Area, and Barycentric Coordinates
  • Vandermonde matrix and determinant
  • Cabart's Collinearity
  • Three Similar Triangles
  • Vandermonde Determinant
  • Divisibility Of Prod-Dif And The Vandermonde Determinant
  • A Case for Determinants
  • A Cyclic Inequality in Three Variables with a Variable Hierarchy

    • |Contact| |Front page| |Contents| |Algebra|

    Copyright © 1996-2018 Alexander Bogomolny

    71924349