Triangle from Angle Bisector, Altitude and Inradius
Construct $\Delta ABC,$ given angle bisector $l_a,$ altitude $h_a,$ and Inradius $r.$
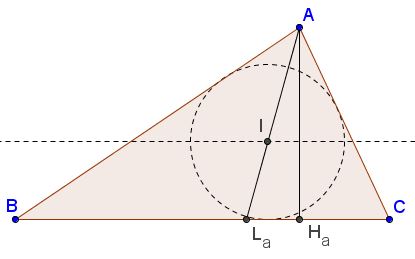
Construction
The construction is straightforward: start with $\Delta AL_{a}H_{a},$ then find the incenter $I$ on $AL_{a}$ at distance $r$ from $L_{a}H_{a}.$ This defines the incircle $(I)$ and the tangents from $A$ that give the vertices $B$ and $C.$
Acknowledgment
The construction was communicated to me by Prof. Dr. René Sperb.
|Contact| |Front page| |Contents| |Up|
Copyright © 1996-2018 Alexander Bogomolny73297415
