Mehmet Sahin's Identity in Triangle
Problem
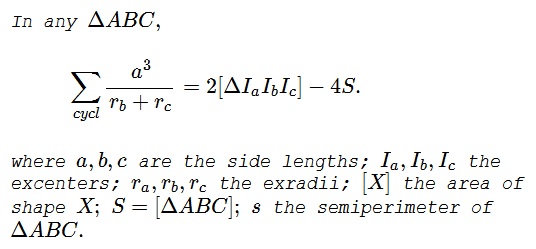
Solution
Below, we are going to use several known identities, $S=rs,$ $S=s_a(s-a),$ $abc=4RS,$ $\displaystyle [\Delta I_aI_bI_c]=\frac{abc}{2r^2s}{S}.$ Thus,
$\displaystyle \begin{align} \sum_{cycl}\frac{a^3}{r_b+r_c} &=\sum_{cycl}\frac{a^3}{\displaystyle \frac{S}{s-b}+\frac{S}{s-c}}\\ &=\frac{1}{S}\sum_{cycl}\frac{a^3(s-b)(s-c)}{2s-a-b}\\ &=\frac{1}{S}\sum_{cycl}a^2(s-b)(s-c)\\ &=\frac{1}{rs}\cdot 4rs^2(R-r)=4s(R-r)=4sR-4sr\\ &=\frac{4Rrs}{r}-4S=\frac{4RS}{r}-4S=2\cdot\frac{abcS}{2r^2s}-4S\\ &=2[\Delta I_aI_bI_c]-4S. \end{align}$
Acknowledgment
The problem from the Romanian Mathematical Magazine has been kindly posted by Dan Sitaru at the CutTheKnotMath facebook page, along with the above solution of his. The problem is by Mehmet Sahin.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73227618
