The Beauty of Fractions
Problem
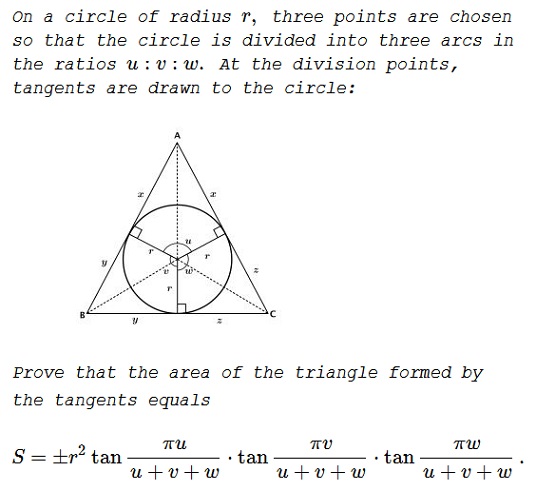
Solution
Set $AB=x+y,\,$ $BC=y+z,\,$ $AC=z+x.\,$ Scale the arc ratios to satisfy $u+v+w=2\pi.\,$ As we can see, $\displaystyle x=r\tan\frac{u}{2},\,$ $\displaystyle y=r\tan\frac{v}{2},\,$ $\displaystyle z=r\tan\frac{w}{2}.\,$
Heron's formula reduces to $S=\sqrt{xyz(x+y+z)},\,$ so we have
$\displaystyle\begin{align} S &= \sqrt{xyz(x+y+z)}\\ &=\sqrt{r^4\left(\sum_{cycl}\tan\frac{u}{2}\right)}\prod_{cycl}\tan\frac{u}{2}\\ &=r^2\sqrt{\left(\prod_{cycl}\tan\frac{u}{2}\right)^2}=\pm r^2\prod_{cycl}\tan\frac{u}{2}\\ &=\pm r^2\tan\frac{\pi u}{u+v+w}\cdot\tan\frac{\pi v}{u+v+w}\cdot\tan\frac{\pi w}{u+v+w}, \end{align}$
where we applied an identity valid for $\alpha+\beta+\gamma=\pi,$
$\tan\alpha+\tan\beta+\tan\gamma=\tan\alpha\cdot\tan\beta\cdot\tan\gamma.$
Acknowledgment
The problem by Francisco Javier García Capitán has been kindly communicated to me by Dan Sitaru, along with his solution.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73296148
