A Parallelogram Proof of the Pythagorean Theorem
John Molokach
22 December, 2010
The proof is based on a dissection of a parallelogram depicted below
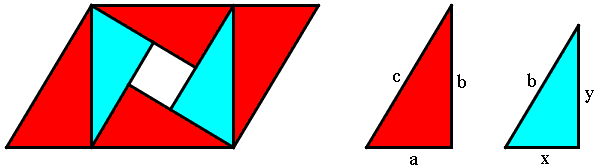
The red right triangle has sides a, b, and the hypotenuse c. The cyan triangle has sides x, y, and the hypotenuse b. The triangles are similar, from which
x = ab/c, y = b²/c.
The area of the parallelogram can be evaluated directly - (a + c)b - and as the sum of the areas of the constituent pieces: 4 red triangles (4·ab/2), 2 cyan triangles (2·xy/2) and a middle rectangle of dimensions (b - x) and (y - a). This we have
(a + c)b = 2ab + xy + (b - x)(y - a) = ab + by + xa.
Now, substitute the values of x and y into the right hand side:
ab + by + xa = ab + b³/c + a²b/c = b(ac + b² + a²)/c,
from which
(a + c)c = ac + b² + a², or c² = a² + b²,
making it one of the algebraic proofs.
This is possible to shorten the proof, making it rather "rectangular" based on a simplified diagram:

|
which leads to
bc = ab + xy + (b - x)(y - a) = by + xa
and more directly to the Pythagorean identity. In this form the proof is a generalization of Proof #3 in the collection.
|Pythagorean Theorem| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73325859
