Vector Algebra in Tetrahedron
Dan Sitaru
28 March, 2016
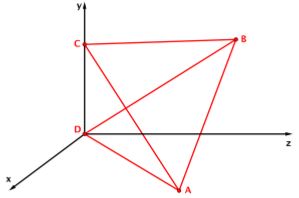
Prove that: $m(\angle (AB,CD)) \ge 90^{\circ}.$
We place the origin at $D\;$ and $(yOz)\equiv(BCD).\;$ It follows that $D=(0,0,0)\;$ and $C=(0,3,0)\;$ because $CD=3.$
Take $B=(0,a,b);\;$ $B\in(yOz),\;$ and $A=(c,d,e),\;$ $a,b,c,d,e\in\mathbb{R}.\;$ Then, via the Pythagorean theorem,
$\begin{cases} BC &= 2\\ AC &= \sqrt{11}\\ BD &= \sqrt{13}\\ AD &= \sqrt{14}\\ AB &= \sqrt{3} \end{cases} \Rightarrow \begin{cases} \sqrt{(a-3)^2+b^2}=2\\ \sqrt{c^2+d^2+e^2}=\sqrt{14}\\ \sqrt{a^2+b^2}=\sqrt{13}\\ \sqrt{c^2+(d-3)^2+e^2}=\sqrt{11}\\ \sqrt{c^2+(d-a)^2+(b-e)^2}=\sqrt{3} \end{cases} $
$ \begin{cases} \sqrt{(a-3)^2+b^2}=2\\ \sqrt{c^2+d^2+e^2}=\sqrt{14}\\ \sqrt{a^2+b^2}=\sqrt{13}\\ \sqrt{c^2+(d-3)^2+e^2}=\sqrt{11}\\ \sqrt{c^2+(d-a)^2+(b-e)^2}=\sqrt{3} \end{cases} \Rightarrow \begin{cases} a^2-6a+9+b^2=4\\ c^2+d^2+e^2=14\\ a^2+b^2=13\\ c^2+(d-3)^2+e^2=11\\ c^2+(d-a)^2+(b-e)^2=3 \end{cases} $
Further,
$ \begin{cases} 13+9-6a=4\;\Rightarrow\;a=3\\ b^2=4\;\Rightarrow\;b=2\\ c^2+d^2-6d+9+e^2=11\;\Rightarrow\;d=2\\ c^2+1+(2-e)^2=3\;\Rightarrow\;e=3,\;c=1. \end{cases} $
Which is to say that $B=(0,3,2)\;$ and $A=(1,2,3).\;$ It follows (in customary notations) that
$\overrightarrow{AB}=-\mathbf{i}+\mathbf{j}-\mathbf{k}\;$ and $\overrightarrow{CD}=-3\mathbf{j}$
so that $\overrightarrow{AB}\cdot\overrightarrow{CD}=-3,\;$ implying $\cos\angle (\overrightarrow{AB},\overrightarrow{CD})\lt 0,\;$ so that $\angle (\overrightarrow{AB},\overrightarrow{CD})\ge 90^{\circ}.$
Remark (by Grégoire Nicollier)
A proof without any computation! Let $C*\;$ and $D*\;$ be the orthogonal projections of $C\;$ and $D\;$ on the line $AB.\;$ The angle between the vectors $AB\;$ and $CD\;$ is obtuse if and only if the vectors $AB\;$ and $C*D*\;$ have opposite directions. This is here clearly the case (without any sketch!) as $\Delta ABC\;$ is obtuse at $B\;$ whereas $\Delta ABD\;$ is almost isosceles.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73235236
