An Angle in Square Conjoint with Regular Pentagon
Problem
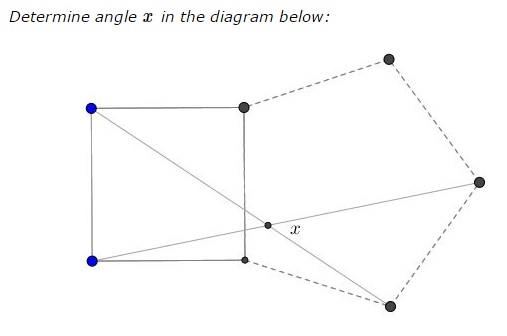
Hint

Solution 1
The proof refers to the diagram below for notations:
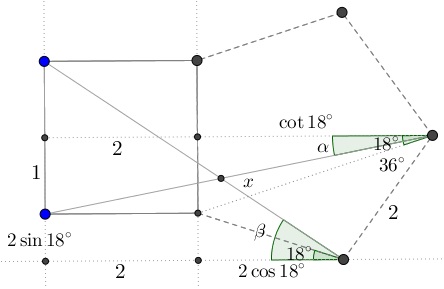
Observe that
- $x = \alpha+\beta.$
- $\displaystyle \tan\alpha=\frac{1}{2+\cot 18^{\circ}}=\frac{\sin 18^{\circ}}{2\sin 18^{\circ}+\cos 18^{\circ}}.$
- $\displaystyle \tan\beta=\frac{1+\sin 18^{\circ}}{1+\cos 18^{\circ}}.$
From these
$\displaystyle \begin{align} \tan x &=\tan (\alpha+\beta) =\frac{\tan\alpha+\tan\beta}{1-\tan\alpha\cdot\tan\beta}\\ &=\frac{\displaystyle \frac{\sin 18^{\circ}}{2\sin 18^{\circ}+\cos 18^{\circ}}+\frac{1+\sin 18^{\circ}}{1+\cos 18^{\circ}}}{\displaystyle 1-\frac{\sin 18^{\circ}}{2\sin 18^{\circ}+\cos 18^{\circ}}\cdot \frac{1+\sin 18^{\circ}}{1+\cos 18^{\circ}}}\\ &=\frac{\displaystyle 2\sin 18^{\circ}\cos 18^{\circ}+\cos 18^{\circ}+\overbrace{(3\sin 18^{\circ}+2\sin^2 18^{\circ})}^{\sqrt{5}/2}}{2\sin 18^{\circ}\cos 18^{\circ}+\cos 18^{\circ}+\underbrace{(\cos 36^{\circ}+\sin 18^{\circ})}_{\sqrt{5}/2}}\\ &=1. \end{align}$
It follows that $x=45^{\circ}.$
Solution 2
Let $C=1,\,$ $E=a,\,$ $F=a^2,\,$ $G=a^3,\,$ $D=a^4,\,$ $a=e^{\frac{2\pi i}{5}}.$ $ABCD\,$ is a positively oriented square. Hence $\displaystyle \frac{B-C}{D-C}=i\,$ and $\displaystyle \frac{A-D}{C-D}=-i,\,$ implying $A=a^4+i(a^4-1)\,$ and $B=1+i(a^4-1),\,$ $\displaystyle \frac{B-G}{A-F}=\frac{BG}{AF}\cdot e^{xi}.$
Thus,
$\displaystyle\begin{align} \frac{B-G}{A-F} &= \frac{1-a^3+i(a^4-1)}{a^4-a^2+i(a^4-1)}\\ &\frac{[1-a^3+i(a^4-1)][a-a^3-i(a-1)]}{|a^4-a^2+i(a^4-1)|^2}\\ &\frac{(2+a-a^3-2a^4)+i(2-2a-a^2+a^4)}{|a^4-a^2+i(a^4-1)|^2}. \end{align}$
Let $z\,$ denotes the numerator of the last faction. We know that $\Re (z)=z+\overline{z}\,$ and $\Im (z)=-i(z-\overline{z}).\,$ In our case,
$\Re (z)=5+i(-3a-a^2+a^3+3a^4)\\ \Im (z)=5+i(-3a-a^2+a^3+3a^4),$
implying $\Re {z}=\Im (z),\,$ and making $\cot x=1\,$ and $x=45^{\circ}.$
Ackowledgment
Leo Giugiuc has kindly posted a link to the Οι Ρομαντικοι της Γεωμετριας (Romantics of Geometry) facebook group. The problem is due to Ruben Dario; Solution 1 is by Rubén Huillca Guevara (Peru); Solution 2 is by Leo Giugiuc.
Halil Ibrahim Ayana has independently came up with the same prolem.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73298782
