Probability of an Odd Number of Sixes

If $0\le k\le n,$ the probability of $k$ sixes turning up in a random toss of $n$ fair dice is $\displaystyle {n\choose k}\left(\frac{5}{6}\right)^{n-k}\left(\frac{1}{6}\right)^{k}.$ Hence, with $\displaystyle a=\frac{5}{6}$ and $\displaystyle b=\frac{1}{6},$ the required probability is

$\displaystyle\begin{align}P&=\sum_{k\text{ is odd}}{n\choose k}\left(\frac{5}{6}\right)^{n-k}\left(\frac{1}{6}\right)^{k}\\ &=\text{ sum of every second term in the expansion of }(a+b)^n\\ &=\frac{1}{2}\left[(a+b)^n-(a-b)^n\right]\\ &=\frac{1}{2}\left[1-\left(\frac{2}{3}\right)^n\right]. \end{align}$

In a similar manner, the probability of an even number of sixes equals

$\displaystyle \begin{align} Q&=\frac{1}{2}\left[(a+b)^n+(a-b)^n\right]\\ &=\frac{1}{2}\left[1+\left(\frac{2}{3}\right)^n\right]. \end{align}$

Naturally, $P+Q=1.$ Why $Q\gt P?$ The graphics below may be suggestive.

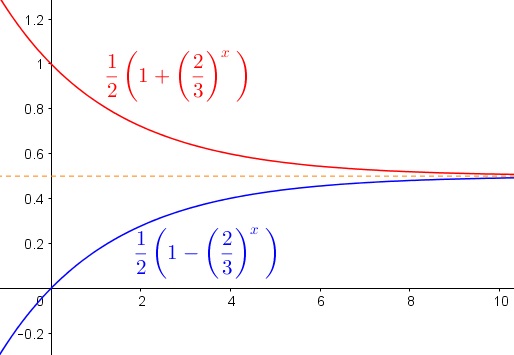
$0$ is an even number always lurking in the background.

This is a slight modification of problem 470 from Five Hundred Mathematical Challenges by E. J. Barbeau et all (MAA, 1995).