Sums of Unit Vectors
Problem
Here's a problem I borrowed from Kunihiko Chikaya's facebook group Enjoy Solving Mathematics (Problem #327):
Given $n\;$ distinct points $A_i,\;$ $i=1,\ldots,n,\;$ on a unit circle with center $O.\;$ The points have the property that, for every $u\in\{1,\ldots,n\},\;$ there are $v,w\in\{1,\ldots,n\}\;$ such that $\overrightarrow{OA_u}=\overrightarrow{OA_v}+\overrightarrow{OA_w}.\;$ Prove that $n\;$ is a multiple of $6.$
Solution
Vector $\overrightarrow{OA_2}\;$ is the sum of two given vectors; for convenience, let $\overrightarrow{OA_u}=\overrightarrow{OA_1}+\overrightarrow{OA_3}:$
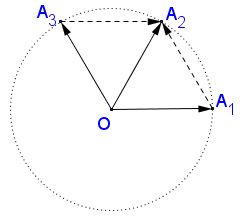
Since $\overrightarrow{OA_u}=\overrightarrow{OA_1}+\overrightarrow{OA_3},\;$ we also have $\overrightarrow{OA_u}=\overrightarrow{OA_1}+\overrightarrow{A_1A_2}$ which informs us that $\Delta OA_1A_2\;$ is quadrilateral and so is $\Delta OA_2A_3.\;$ In general, assuming that that the points $A_v,\;$ $A_u,\;$ $A_w\;$ are set on circle $(O)\;$ in the order, $\overrightarrow{OA_u}=\overrightarrow{OA_v}+\overrightarrow{OA_w}\;$ implies that $\angle A_vOA_u=\angle A_uOA_w=60^{\circ}\;$ and that $OA_u\;$ bisects $\angle A_vOA_w.$
Vector $\overrightarrow{OA_3}\;$ is also the sum of two vectors $\overrightarrow{OA_i}\;$ and, as a consequence of the preceding paragraph, one of these vectors is necessarily $\overrightarrow{OA_2};\;$ denote the other $\overrightarrow{OA_4}.\;$ Naturally, $\angle A_3OA_4=60^{\circ}.\;$ In this manner we also identify points $A_5\;$ and $A_6\;$ and verify that $\angle A_6OA_1=60^{\circ}\;$ and that $\overrightarrow{OA_1}=\overrightarrow{OA_6}+\overrightarrow{OA_2}.$

If $n\gt 6,\;$ then none of the first six vectors appears in $\overrightarrow{OA_u}=\overrightarrow{OA_v}+\overrightarrow{OA_w},\;$ provided that is true for $\overrightarrow{OA_u}\;$ and we are thus led to additional six points. We therefore count $n\;$ by $6s,\;$ which shows that $n\;$ is divisible by $6.$
Extension problems
The problem can be modified to insure that $n\;$ is a multiple of other integers. For example,
Given $n\;$ distinct points $A_i,\;$ $i=1,\ldots,n,\;$ on a unit circle with center $O.\;$ The points have the property that, for every $u\in\{1,\ldots,n\},\;$ there are $v,w\in\{1,\ldots,n\}\;$ such that
$t\cdot\overrightarrow{OA_u}=\overrightarrow{OA_v}+\overrightarrow{OA_w}.\;$
For a real positive $t.\;$ Prove that, for $\displaystyle t\in\{\sqrt{2},\sqrt{3},\varphi,\frac{1}{\varphi},\frac{\sqrt{3}+1}{\sqrt{2}},\frac{\sqrt{3}-1}{\sqrt{2}}\}\;$ where $\varphi\;$ is the Golden Ratio, $n\;$ is a multiple of $8,\;$ $12,\;$ $10,\;$ $5,\;$ $24,\;$ and $24\space(!),\;$ respectively.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73299526
