Sanchez's Areas in Bottema's Configuration
Source
Leo Giugiuc has kindly posted at the CutTheKnotMath facebook page an exciting problem by Miguel Ochoa Sanchez:
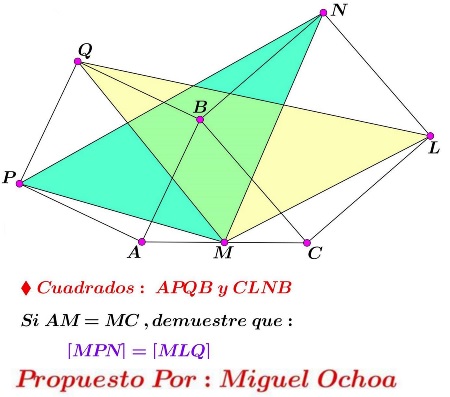
Problem
$ABQP$ and $CBNL$ are two squares sharing a vertex. $M$ is the midpoint of $AC.$
Prove that $[\Delta MPN]=[\Delta MLQ],$ where $[F]\;$ denotes the area of shape $F.$
Solution 1
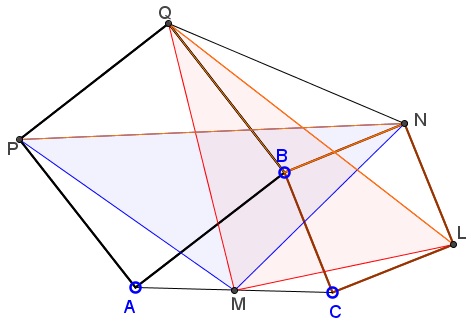
Note that $\Delta MPN=\Delta BPN\cup\Delta MBP\cup\Delta MBN\;$ and $\Delta MLQ=\Delta BLQ\cup\Delta MBL\cup\Delta MBQ:$

Let's set $BC=c,\;AB=a.\;$ Observe that $\angle PBN=QBN+45^{\circ}=\angle QBL.\;$ Denote this angle as $\omega.\;$ Then
$[\Delta BPN]=\frac{1}{2}(a\sqrt{2})\cdot c\cdot\sin\omega=\frac{1}{2}a\cdot (c\sqrt{2})\cdot\sin\omega=[\Delta BLQ].$
Introduce angles $\alpha$ and $\gamma$ as shown:

Then, since $[\Delta ABM]=[\Delta CBM],$
$a\sin\alpha=c\sin\gamma.$
Using that,
$\begin{align} 2([\Delta MBP]+[\Delta MBN]) &= BM\cdot (BP\cdot\sin \angle MBP+BN\cdot\sin\angle MBN)\\ &= BM\cdot (a\sqrt{2}\sin (\alpha+45^{\circ})+c\cdot\sin (\gamma+90^{\circ}))\\ &= BM\cdot (a\sin\alpha+a\cos\alpha+c\cos\gamma)\\ &= BM\cdot (c\sin\gamma+a\cos\alpha+c\cos\gamma)\\ &= BM\cdot (a\cos\alpha+c\sin\gamma+c\cos\gamma)\\ &= BM\cdot (a\sin (\alpha+90^{\circ})+c\sqrt{2}\sin(\gamma+45^{\circ}))\\ &= BM\cdot (BQ\cdot\sin\angle MBQ+BL\cdot\sin\angle MBL)\\ &= 2(\Delta MBQ]+[\Delta MBL]), \end{align}$
which proves the required $[\Delta MPN]=[\Delta MLQ].$
Solution 2
This solution is by Gréoire Nicollier.
Proof with complex numbers. Take $A=-1,\;$ $B=z,\;$ $C=1.\;$ Then $L=1-i(z-1),\;$ $N=z-i(z-1),\;$ $P=-1+i(z+1),\;$ $Q=P=z+i(z+1).\;$ $Area(0,U,V)=|Im(UV^*)|/2,\;$ where $V^*\;$ is the conjugate of $V.\;$ A short calculation gives $Im(PN^*)=Im(QL^*) = |z|^2 + 3 Im(z) + 1.$
|Up| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny
73293847
