A Problem with Right Isosceles Triangles

Source

Leo Giugiuc has kindly posted at the CutTheKnotMath facebook page another problem by Miguel Ochoa Sanchez:

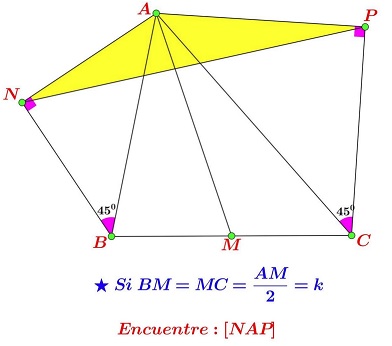
A Problem with Right Isosceles Triangles - source

Problem

The configuration below has the following properties:

$BM=CM=AM/2,$
$\angle ABN=\angle ACP=45^{\circ},$
$\angle ANB=\angle APC=90^{\circ}.$

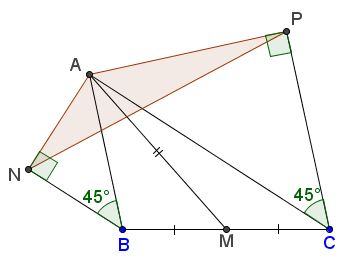
A Problem with Right Isosceles Triangles - problem

Express the area $[\Delta NAP]$ in terms of $k=BM.$

Solution

Denote $\alpha =\angle BAC.$ Then, since triangles $ABN$ and $ACP$ are right isosceles, $\angle NAP =\alpha +90^{\circ}.$ It follows that

$\displaystyle\begin{align} 2[NAP] &= AN\cdot AP\cdot\sin (\alpha +90^{\circ})\\ &=\frac{AB}{\sqrt{2}}\cdot\frac{AC}{\sqrt{2}}\cdot\cos\alpha\\ &=\frac{1}{2}AB\cdot AC\cdot\cos\alpha. \end{align}$

So that $4[NAP]=AB\cdot AC\cdot\cos\alpha.$

By the Law of Cosines,

$\begin{align} BC^{2}&=AB^{2}+AC^{2}-2AB\cdot AC\cdot\cos\alpha\\ &=AB^{2}+AC^{2}-8[NAP]. \end{align}$

On the other hand, $AM$ is a median in $\Delta ABC$ and, as is well known, $\displaystyle AM^2=\frac{AB^2+AC^2}{2}-\frac{BC^2}{4}$ from which (since $AM=BC=2k)$ $AB^2+AC^2=10k^2.$ A substitution then gives $4k^2=BC^2=10k^2-8[NAP].$ In other words, $\displaystyle [NAP]=\frac{3}{4}k^2.$

71923799