Miguel's Tangents: Modern Day Sangaku
What Might This Be About?
Source
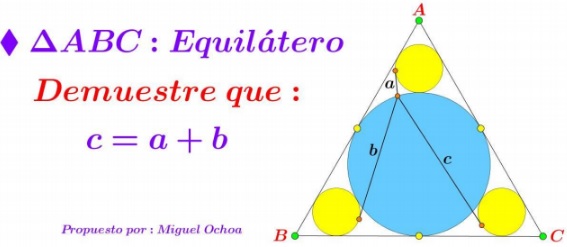
Problem
$ABC$ is an equilateral triangle, with the incircle $(O).\;$ Circles $(O_a),\;$ $(O_b),\;$ $(O_c)\;$ are inscribed into the angles at $A,B,C,\;$ respectively, tangent to $(O).\;$ From point $P\;$ on $(O)\;$ tangents of lengths $a,b,c\;$ are drawn to the circles $(O_a),\;$ $(O_b),\;$ $(O_c).\;$
Assuming that $c\ge a\;$ and $c\ge b,\;$ prove that $c=a+b.$
Solution
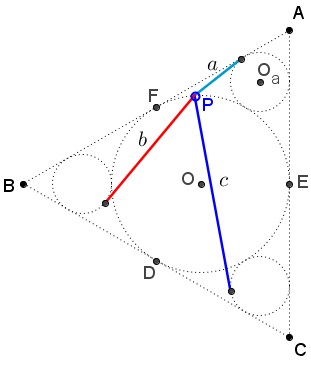
Assume the incircle $(O)\;$ is defined by the equation $|z|=1\;$ and that the points of tangency are $D=u^2\;,$ $E=1,\;$ $F=u,\;$ where $\displaystyle u=-\frac{1}{2}+i\frac{\sqrt{3}}{2}.\;$ We get the system
$\begin{cases} B+C=2u^2 &\\ A+B=2u &\\ C+A=1 & \end{cases}$
from which $A=-2u^2,\;$ $B=-2,\;$ and $C=-2u.\;$ In other words, $A=(1,\sqrt{3}),\;$ $B=(-2,0),\;$ $C=(1,-\sqrt{3}).$
Circles $(O_a),\;$ $(O_b),\;$ $(O_c)\;$ are known to have the radius $\frac{1}{3},\;$ so that $\displaystyle O_a=\left(\frac{2}{3},\frac{2}{\sqrt{3}}\right),\;$ $\displaystyle O_b=\left(-\frac{4}{3},0\right),\;$ and $\displaystyle O_c=\left(\frac{2}{3},-\frac{2}{\sqrt{3}}\right).\;$
We chose $P=(\cos t,\sin t),\;$ $\displaystyle\frac{\pi}{3}\le t\le \pi.$ Thus we may compute $\displaystyle a=\sqrt{O_aP^2-\frac{1}{9}}=\frac{4}{\sqrt{3}}\sin\left(\frac{t}{2}-\frac{\pi}{6}\right),\;$ $\displaystyle b=\frac{4}{\sqrt{3}}\cos\frac{t}{2},\;$ $\displaystyle c=\frac{4}{\sqrt{3}}\sin\left(\frac{t}{2}+\frac{\pi}{6}\right),\;$ and, since $\displaystyle \sin\left(\frac{t}{2}+\frac{\pi}{6}\right)-\sin\left(\frac{t}{2}-\frac{\pi}{6}\right)=\cos\frac{t}{2},\;$ we, obviously have $c=a+b.$
Acknowledgment
The above problem, due to Miguel Ochoa Sanchez, has been communicated to me by Leo Giugiuc, along with his solution.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2015 Alexander Bogomolny73299695
