Collinear Intersections and Products of Ratios
What Might This Be About?
Problem
For an integer $n\ge 2$ and two sets of points $\{A_{i}: i=1,\ldots ,n\}$ and $\{B_{i}: i=1,\ldots ,n\},$ define $C_{i}=A_{i}A_{i+1}\cap B_{i}B_{i+1},$ $ i=1,\ldots ,n-1,$ $C_{n}=A_{n}B_{1}\cap A_{1}B_{n}.$
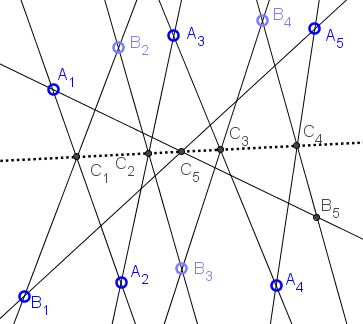
Prove that if $C_i,\space i=1,\ldots ,n,$ are collinear then
$\frac{A_1C_1}{A_2C_1}\cdot\frac{A_2C_2}{A_3C_2}\cdots\frac{A_nC_n}{A_1C_n}\cdot\frac{B_1C_1}{B_2C_1}\cdot\frac{B_2C_2}{B_3C_2}\cdots\frac{B_nC_n}{B_1C_n}=1.$
Solution
Solution is wanting.
Application
Let four points $A,$ $B,$ $C,$ $D$ lie on a plane. $AB$ meets $CD$ at $F;$ $AC$ meets $DB$ at $E.$
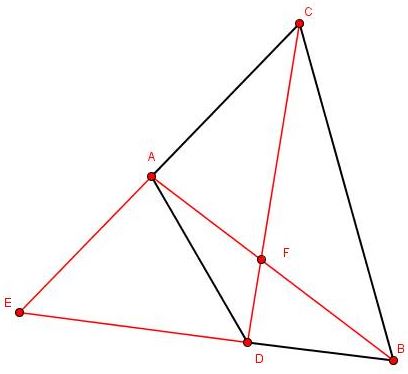
Then the following identity holds:
$\displaystyle\frac{FA}{FB}\cdot \frac{EB}{ED}\cdot \frac{FD}{FC}\cdot \frac{EC}{EA}=1.$
Acknowledgment
The problem has been posted by Dao Thanh Oai (Vietnam) at the CutTheKnotMath facebook page.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73292942
