Equal And Perpendicular Segments in a Square
What Is This About?
Problem
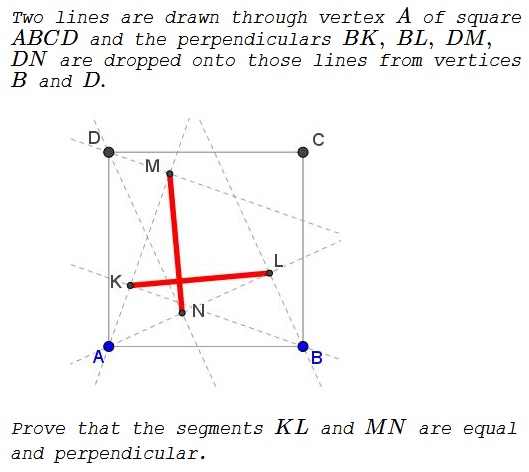
Solution 1
Assuming $A=(0,0),$ $B=(1,0),$ $D=(0,1),$ and that the two lines are defined by the equations $y=ax$ and $y=bx,$ we find
$\displaystyle\begin{array}{cc} K=\left(\frac{1}{b^2+1},\frac{b}{b^2+1}\right)&L=\left(\frac{1}{a^2+1},\frac{a}{a^2+1}\right)\\ M=\left(\frac{b}{b^2+1},\frac{b^2}{b^2+1}\right)&L=\left(\frac{a}{a^2+1},\frac{a^2}{a^2+1}\right)\\ KL=\frac{|a-b|}{\sqrt{(a^2+1)(b^2+1)}}&MN=\frac{|a-b|}{\sqrt{(a^2+1)(b^2+1)}}\\ \text{slope of KL }=\frac{ab-1}{a+b}&\text{slope of MN }=\frac{a+b}{1-ab} \end{array}$
Solution 2
Consider a clockwise rotation $\tau$ through $90^{\circ}$ around the center of the square so that $\tau(B)=A$ and $\tau(A)=D.$
Note that $\angle KBA=90^{\circ}-\angle KAB=\angle KAD$ and, similarly, $\angle KAB=\angle ADM.$ It follows that $\tau(\overset{\leftrightarrow}{BK})=\overset{\leftrightarrow}{AM}$ and $\tau(\overset{\leftrightarrow}{AK})=\overset{\leftrightarrow}{DM}.$ Thus, $\tau(K)=\tau(\overset{\leftrightarrow}{BK}\cap\overset{\leftrightarrow}{AK})=\overset{\leftrightarrow}{AM}\cap\overset{\leftrightarrow}{DM}=M.$ By the same token $\tau(L)=N.$
Acknowledgment
This is problem M1202 from the Russian Kvant magazine (1990). The problem is by D. Nyamsuren, Solution 2 is by V. Dubrovsky.
![]()
|Contact| |Up| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73299815
