Regular Hexagon and Triangle Joined at Vertex
What Might This Be About?
Problem
A regular hexagon and an equilateral triangle are joined at vertex $B.$ Vertices $A$ and $A'$ are adjacent to $B,$ one on the hexagon, the other on the triangle. Let $O$ be the center of the hexagon, $Q$ the center of the triangle, $M'$ the midpoint of $AA'.$
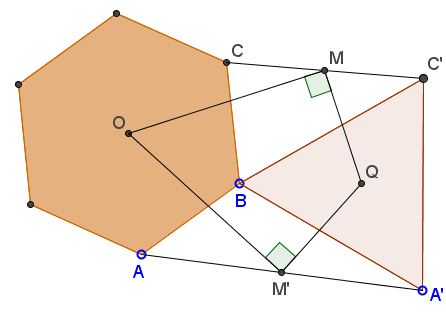
Then $\angle OM'Q=90^{\circ}.$
Solution 1
This is a particular case of the generalized Bottema's theorem.
Solution 2
Let $P$ and $N$ be the midpoints of $AB$ and $A'B,$ respectively.
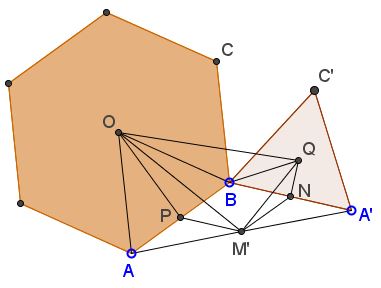
$\begin{align} \angle OPM' &= \angle OPB + \angle BPM'\\ &=90^{\circ}+ (180^{\circ}-\angle PBN = 270^{\circ}-\angle PBN\\ &=360^{\circ}-(60^{\circ}+30^{\circ}+\angle PBN)\\ &=360^{\circ}-(\angle OPB+\angle NBQ^{\circ}+\angle PBN)\\ &=\angle OBQ. \end{align}$
Similarly, $\angle M'NQ=\angle OBQ.$ Further, triangles, $BOP$ and $QBN$ are similar (both are $30-90-60).$ Thus we have a proportion $OP/OB=BN/BQ$ so that $OP/BN=OB/BQ,$ but $BN=PM'$ which, together with $\angle OPM'=\angle OBQ,$ shows that triangles $OPM'$ and $OBQ$ are similar. In the same manner, $\Delta M'NQ\sim\Delta OBQ.$ All this combines to show the following proportions:
$\displaystyle\frac{OP}{OM'}=\frac{M'N}{M'Q}=\frac{BP}{M'Q},$ i.e., $\displaystyle\frac{OP}{BP}=\frac{OM'}{M'Q}.$
and also
$\displaystyle\frac{OP}{OM'}=\frac{OB}{OQ}.$
It follows that $\Delta OBP\sim\Delta OQM',$ such that $\angle OM'Q=\angle OBP=90^{\circ}.$
Solution 3
With apologies, the notations below are somewhat are different from the ones above. Hopefully, this does not make the proof unnecessarily confusing.
Let $E$ and $D$ be the reflections in $M$ of $O_1$ and $B,$ respectively.

Note that triangles $OAE$ and $OBO_1$ are congruent (SAS), implying $OE=OO_1.$ Then, since $AM=MC,$ $O_1M=ME;$ so in $Delta EOO_1$ $OM$ is an altitude, hence, $\angle OMO_1=90^{\circ}.$
Acknowledgment
The problem has been posted by Leo Giugiuc (Romania) at the Short Mathematical Idea facebook page in the name of Miguel Ochoa Sanchez (Peru); Solution 2 is due to Stan Fulger (Romania); solution 3 is by Miguel Ochoa Sanchez.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73300644
