Cyclic Quadrilateral, Angle Bisector And Isosceles Triangle
What Is This About?
Problem
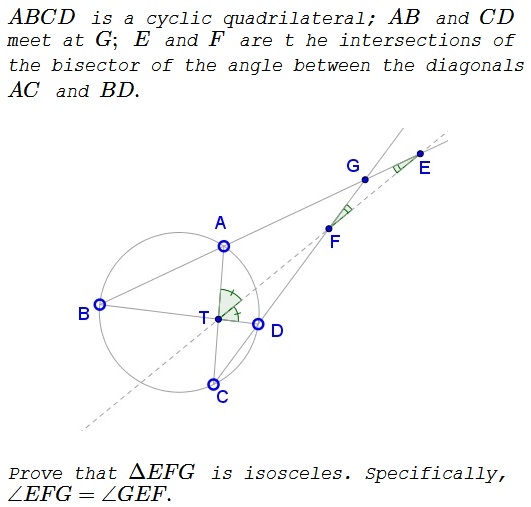
Solution 1
$\angle BAC=\angle BDC\,$ as inscribed into the same circle and subtended by the same arc. $\angle CAG=\angle BDF\,$ as supplementary to two equal angles.
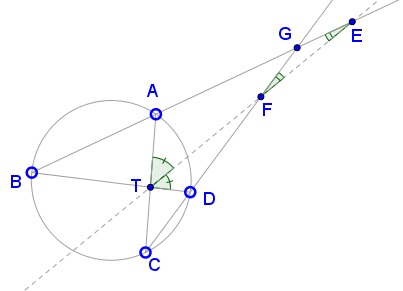
Let $T\,$ be the intersection of the diagonals of the quadrilateral. In triangles $ATE\,$ and $DTF,\,$ $\angle ATE=\angle DTF\,$ and $\angle EAT=\angle FDT,\,$ implying that $\angle AET=\angle DFT.\,$ But the latter is vertical with $\angle EFG,\,$ prving $\angle EFG=\angle GEF,\,$ as required.
Solution 2
Let $K\,$ and $L\,$ be the intersections of the angle bisector with the circle, $T\,$ the intersection of the diagonals:
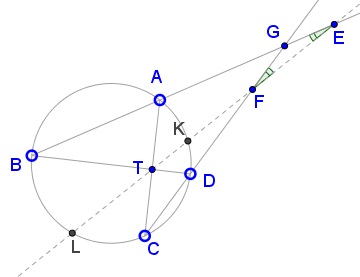
Then
$\displaystyle\begin{align} \frac{1}{2}\left(\overset{\frown}{AK}+\overset{\frown}{CL}\right)&=\angle ATK=\angle DTK\\ &=\frac{1}{2}\left(\overset{\frown}{DK}+\overset{\frown}{BL}\right). \end{align}$
On the other hand,
$\displaystyle\begin{align} \angle BEL &=\frac{1}{2}\left(\overset{\frown}{BL}-\overset{\frown}{AK}\right)\,\text{and}\\ \angle LFC &=\frac{1}{2}\left(\overset{\frown}{CL}-\overset{\frown}{DK}\right), \end{align}$
making $\angle EFG=\angle LFC=\angle GEF.$
Solution 3
Let,
$\displaystyle \begin{align} &\angle ABD = \angle ACD = \alpha, ~\text{(inscribed angles)} \\ &\angle BAC = \angle BDC = \beta. \end{align}$
Note,
$\displaystyle \begin{align} \angle TDF &= \pi-\angle BDC = \pi-\beta,~\text{(supplementary angles)} \\ \angle ATD &= \alpha+\beta.~\text{(exterior angle)} \end{align}$
Choose the positive $X$ axis from $B$ to $D$. Thus,
$\displaystyle \begin{align} \hat{BA}&=\cos\alpha\hat{x}+\sin\alpha\hat{y}, \\ \hat{TF}&=\cos\left(\frac{\alpha+\beta}{2}\right)\hat{x}+\sin\left(\frac{\alpha+\beta}{2}\right)\hat{y}, \\ \hat{DF}&=-\cos(\pi-\beta)\hat{x}+\sin(\pi-\beta)\hat{y} =\cos\beta\hat{x}+\sin\beta\hat{y}, \end{align}$
where $\hat{}$ represents a unit vector. Thus,
$\displaystyle \begin{align} &\hat{BA}\cdot\hat{TF}-\hat{DF}\cdot\hat{TF} \\ &=(\cos\alpha-\cos\beta)\cos\left(\frac{\alpha+\beta}{2}\right) +(\sin\alpha-\sin\beta) \sin\left(\frac{\alpha+\beta}{2}\right) \\ &=\left[-2\sin\left(\frac{\alpha-\beta}{2}\right)\sin\left(\frac{\alpha+\beta}{2}\right)\right] \cos\left(\frac{\alpha+\beta}{2}\right) \\ &+\left[2 \sin\left(\frac{\alpha-\beta}{2}\right) \cos\left(\frac{\alpha+\beta}{2}\right)\right] \sin\left(\frac{\alpha+\beta}{2}\right) \\ &= 0 \end{align}$
Thus, the angle between $BA$ and $TF$ ($\angle GEF$) is the same as the angle between $DF$ and $TF$ ($\angle GFE$).
Acknowledgment
This problem has been kindly posted at the CutTheKnotMath facebook page by Bo Cua Ga. The problem provides a natural platform for angle chasing. Solution 3 is by Amit Itagi.
![]()
|Contact| |Up| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73300798
