Triangle Area in Square with Incircle
Source
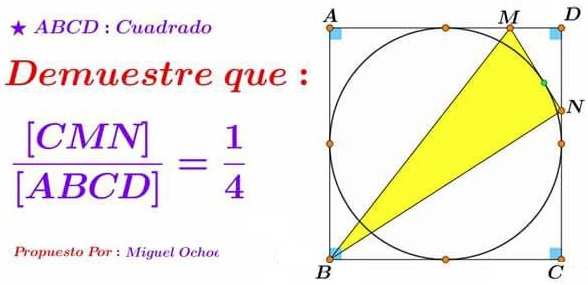
Problem
Solution 1
WLOG, let the side length of the square be $2,\,$ with the center at the origin. Let $T=(\cos\alpha, \sin\alpha),\,$ $(0\lt\alpha\lt\frac{\pi}{2})\,$ be the point of tangency, we have the equation of the tangent line of the circle : $x\cos\alpha + y\sin\alpha = 1,\,$ thus we get $\displaystyle M=(\frac{1 - \sin\alpha}{\cos\alpha}, 1)\,$ and $\displaystyle N=(1, \frac{1 -\cos\alpha}{\sin\alpha}),\,$ yielding vector $\displaystyle \overset{\rightarrow}{BM} = \left(\frac{1 - \sin\alpha + \cos\alpha}{\cos\alpha}, 2\right)\,$ and vector $\displaystyle \overset{\rightarrow}{BN} = \left(2, \frac{1 - \cos\alpha + \sin\alpha}{\sin\alpha}\right),\,$ therefore using the formula of the area of a triangle with vertices $O(0,0),\,$ $P(a, b),\,$ $Q(c, d)\,$ which is $[\Delta OPQ] = \displaystyle \frac{1}{2}|ad - bc|,\,$ we obtain $\displaystyle [\Delta BMN] = \frac{1}{2}\left|\frac{(1 - \sin\alpha + \cos\alpha)(1 - \cos\alpha + \sin\alpha)}{\sin\alpha\cos\alpha} - 2\times 2\right| = 1,\,$ yielding $\displaystyle \frac{[\Delta BMN]}{[ABCD]} = \frac{1}{4}.$
Solution 2
Let $P,Q\,$ be the tangency points, as below:

Set $PM=PT=x\,$ and $TN=NQ=y.\,$ Then, from $MD^2+DN^2=MN^2,\,$ i.e., $\displaystyle\left(\frac{1}{2}-x\right)^2+\left(\frac{1}{2}-y\right)^2=(x+y)^2,\,$ we get $1-2x-2y-4xy=0.$
Further,
$\displaystyle \begin{align} [\Delta BMN]&=[ABCD]-[\Delta ABM]-[\Delta BMN]-[\Delta NBC]\\ &=\small{1-\frac{\displaystyle 1\cdot\left(\frac{1}{2}+x\right)}{2}-\frac{\displaystyle 1\cdot\left(\frac{1}{2}-x\right)\left(\frac{1}{2}-y\right)}{2}-\frac{\displaystyle 1\cdot\left(\frac{1}{2}+y\right)}{2}}\\ &=1-\frac{1}{4}-\frac{x}{2}-\frac{1}{8}+\frac{x+y}{4}-\frac{xy}{2}-\frac{1}{4}-\frac{y}{2}\\ &=\frac{3}{8}-\frac{x+y}{4}-\frac{xy}{2}=\frac{3-2(x+y)-4xy}{8}\\ &=\frac{2+0}{8}=\frac{1}{4}. \end{align}$
Solution 3
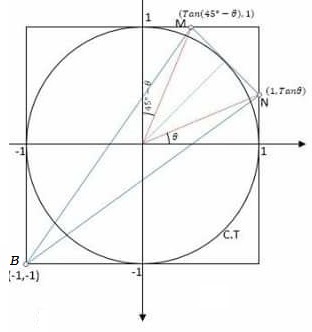
$\displaystyle\begin{align} [ABCD]&=4\\ [\Delta BMN]&=\frac{1}{2}\left|\begin{array}{cc}2 & \tan\theta+1\\\tan (45^{\circ}-\theta)+1&2\end{array}\right|\\ &=\frac{1}{2}(3-\underbrace{(\tan\theta+\tan (45^{\circ}-\theta)+\tan\theta\cdot\tan (45^{\circ}-\theta))}_{1}\\ &=1. \end{align}$
It follows that $\displaystyle \frac{[\Delta BMN]}{[ABCD]}=\frac{1}{4}.$
Solution 4
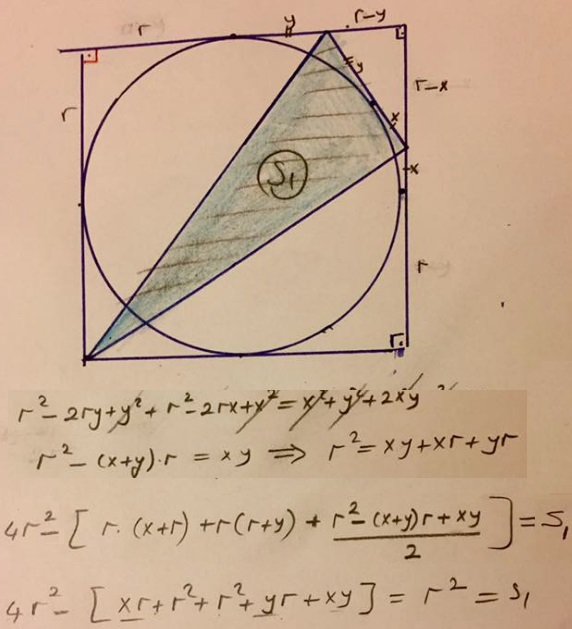
Acknowledgment
The problem has been posted at the CutTheKnotMath facebook page by Miguel Ochoa Sanchez. Solution 1 is by Kunihiko Chikaya; Solution 2 is by Marian Dinca; Solution 3 is by Gabriel Ruddy Cruz Mendéz; Solution 4 is by Bariş Altay.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73298290
