An Appearance of a 30-60-90 Triangle
What Is This About?
Problem
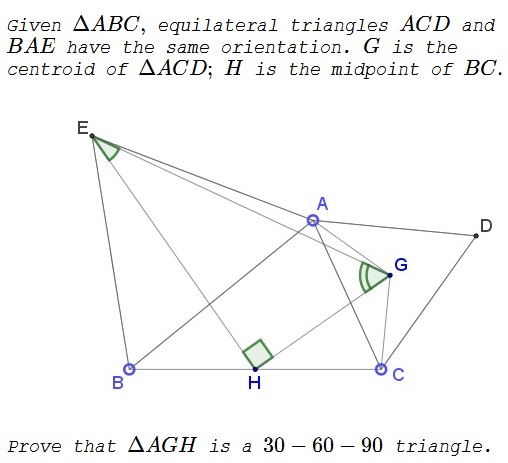
Solution 1
Let $J$ be the midpoint of $AC.$ We shall prove that triangles $GJH$ and $GAE$ are similar and the latter is twice as big as the former.
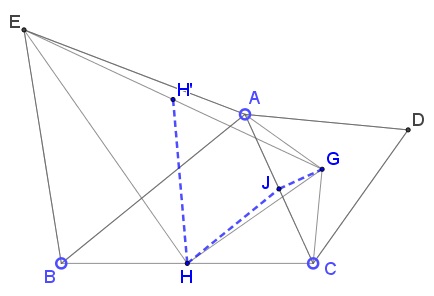
First, $\Delta AGJ$ is $30-60-90$ such that $AG=2GJ.$ Second, since $J$ and $H$ are the midpoints of the sides $AC$ and $BC,$ $AE=AB=2HJ.$ Third,
$\begin{align} \angle EAG&=60^{\circ}+\angle BAC+30^{\circ}=\angle BAC+90^{\circ}\\ \angle HJG&=\angle HJC+90^{\circ}=\angle BAC+90^{\circ}. \end{align}$
If $H'$ is the midpoint of $EG,$ then $\Delta GHH'$ is equilateral, whereas $\Delta EH'H$ is isosceles with $\angle EH'H=120^{\circ},$ making base angles equal $30^{\circ}$ so that $\angle EHG=90^{\circ}.$
Solution 2
Let $\hat{z}$ be a unit vector pointing out of the plane. Let $\vec{AB}=\vec{p}$ and $\vec{AC}=\vec{q}$. Let $\vec{u}=\hat{z}\times\vec{p}$ and $\vec{v}=\hat{z}\times\vec{q}$. Note, $\vec{u}\cdot\vec{p}=0$, $\vec{u}\cdot\vec{q}=0$, $\vec{v}\cdot\vec{p}=0$, $\vec{v}\cdot\vec{q}=0$, $\hat{u}\times\vec{z}=\vec{p}$. Also,
$\displaystyle \vec{u}\cdot\vec{v}=\left(\hat{z}\times\vec{p}\right)\cdot\left(\hat{z}\times\vec{q}\right)=\vec{p}\cdot\vec{q}.$
Writing out the other vectors,
$\displaystyle \begin{align} \vec{AE}&=\frac{1}{2}\vec{p}-\frac{\sqrt{3}}{2}\vec{u},~\vec{AD}=\frac{1}{2}\vec{q}+\frac{\sqrt{3}}{2}\vec{v},~\vec{AH}=\frac{1}{2}\vec{p}+\frac{1}{2}\vec{q} \\ \vec{AG}&=\frac{1}{3}\vec{AC}+\frac{1}{3}\vec{AD} =\frac{1}{2}\vec{q}+\frac{1}{2\sqrt{3}}\vec{v}. \end{align}$
$\displaystyle \begin{align} \vec{HG}\cdot\vec{HE} &=\left(\vec{AG}-\vec{AH}\right)\cdot \left(\vec{AE}-\vec{AH}\right) =\left(\frac{1}{2\sqrt{3}}\vec{v}-\frac{1}{2}\vec{p}\right)\cdot \left(-\frac{\sqrt{3}}{2}\vec{u}-\frac{1}{2}\vec{q}\right) \\ &=\frac{1}{4}\left(\vec{p}\cdot\vec{q}-\vec{u}\cdot\vec{v}\right) =\frac{1}{4}\left(\vec{p}\cdot\vec{q}-\vec{p}\cdot\vec{q}\right)=0 \end{align}$
Hence, $\angle EHG=90^{\circ}$.
$\displaystyle\begin{align} \vec{HE}\times\hat{z} &=\left(-\frac{\sqrt{3}}{2}\vec{u}-\frac{1}{2}\vec{q}\right) \times\hat{z} =\left(-\frac{\sqrt{3}}{2}\vec{p}+\frac{1}{2}\vec{v}\right) =\sqrt{3}\left(\frac{1}{2\sqrt{3}}\vec{v}-\frac{1}{2}\vec{p}\right)\\ &=\sqrt{3}\vec{HG}. \end{align}$
Hence, $HE=HG\tan~60^{\circ}$ and $\Delta EHG$ is $30-60-90$.
Acknowledgment
This is a problem from R. Honsberger's Mathematical Chestnuts from Around the World (MAA, 2001, pp 66-68.) The problem has originally appeared in the $\pi\mu\epsilon$ magazine (1984, #553, proposed by Jack Garfunkel and solved by Peter John Dombrowsky.) Solution 2 is by Amit Itagi.
![]()
|Contact| |Up| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73294144
