Completing the Square Where It Matters
Problem
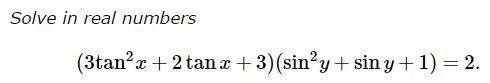
Solution
$\displaystyle 3\tan^2x+2\tan x+3=3\left(\tan x+\frac{1}{3}\right)^2+\frac{8}{3},\\ \displaystyle \sin^2y+\sin y+1=\left(\sin y+\frac{1}{2}\right)^2+\frac{3}{4}.$
Thus the problem becomes
$\displaystyle \left[3\left(\tan x+\frac{1}{3}\right)^2+\frac{8}{3}\right]\cdot \left[\left(\sin y+\frac{1}{2}\right)^2+\frac{3}{4}\right]=2.$
This reduces to
$\displaystyle 3\left(\tan x+\frac{1}{3}\right)^2\left(\sin y+\frac{1}{2}\right)^2+\frac{9}{4}\left(\tan x+\frac{1}{3}\right)^2+\frac{8}{3}\left(\sin y+\frac{1}{2}\right)^2=0,$
where in the left-hand side all the terms are non-negative, meaning that necessarily
$\displaystyle \sin y=-\frac{1}{2}\;\mbox{and}\;\tan y=-\frac{1}{3}.$
In other words,
$\displaystyle y=(-1)^{k+1}\frac{\pi}{6}+k\pi,\;k\in\mathbb{Z},\\ \displaystyle x=-\arctan\frac{1}{3}+m\pi,\;m\in\mathbb{Z}.$
Acknowledgment
The problem, with the above solution, has been posted at the CutTheKnotMath facebook page by Dan Sitaru. The problem is from Dan's book "Math Accent".
|Up| |Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny
73298376
