A Fancy Sum from the APMO 2000,
Problem 1
Problem
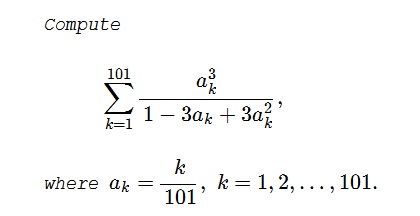
Solution 1
Note that $x^3-3x^2y+3xy^2=(x-y)^3+y^3.$ Thus, multiplying out, the sum at hand becomes to
$\displaystyle \begin{align} \sum_{k=1}^{101}\frac{a_k^3}{1-3a_k+3a_k^2}&=\sum_{k=1}^{101}\frac{k^3}{(101-k)^3+k^3}=\sum_{k=0}^{101}\frac{k^3}{(101-k)^3+k^3}\\ &=\sum_{k=0}^{101}\frac{(101-k)^3}{k^3+(101-k)^3}\\ &=\sum_{k=0}^{50}\frac{k^3+(101-k)^3}{k^3+(101-k)^3}=51. \end{align}$
Solution 2
Rewrite the summand as:
$\displaystyle f (k)=\frac{k^3}{3 k^2 N-3 k N^2+N^3}$
We have $f(N)=1$ and
$f(1)=1-f(N-1)\\ f(2)=1-f(N-2)\\ \cdots\\ f(k)=1-f(N-k)$
Hence
$\displaystyle \sum _{k=1}^N \frac{k^3}{3 k^2 N-3 k N^2+N^3}=\frac{N-1}{2}+1$With $N=101$, the sum equals $51$.
Solution 3
Let $a_0=1-a_{101}=0$.
$\displaystyle \begin{align} S&=\sum_{k=1}^{101}\frac{a_k^3}{1-3a_k+3a_k^2} =\sum_{k=1}^{101}\frac{a_k^3}{a_k^3+(1-a_k)^3} =\sum_{k=1}^{101}\frac{a_k^3}{a_k^3+a_{101-k}^3} \\ &=\sum_{k=1}^{50}\frac{a_k^3+a_{101-k}^3}{a_k^3+a_{101-k}^3} +\frac{a_{101}^3}{a_{101}^3+a_{0}^3} =51. \end{align}$
Acknowledgment
This problem was posted by Leo Giugiuc at the CutTheKnotMath facebook page, along with a solution of his. This was followed by Nguyen Viet Hung's comment to the effect that that's Problem 1 from the $12^{th}$ APMO (2000).
Solution 2 is by N. N. Taleb; Solution 3 is by Amit Itagi.
![]()
|Contact| |Up| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73302284
