TRIGONOMETRIC
Delights

Preface

There is perhaps nothing which so occupies the middle
position of mathematics as trigonometry.
-J. F. HERBART (1890)

This book is neither a textbook of trigonometry - of which there are many - nor a comprehensive history of the subject, of which there is almost none. It is an attempt to present selected topics in trigonometry from a historic point of view and to show their relevance to other sciences. It grew out of my love affair with the subject, but also out of my frustration at the way it is being taught in our colleges.

First, the love affair. In the junior year of my high school we were fortunate to have an excellent teacher, a young, vigorous man who taught us both mathematics and physics. He was a no-nonsense teacher, and a very demanding one. He would not tolerate your arriving late to class or missing an exam-and you better made sure you didn't, lest it was reflected on your report card. Worse would come if you failed to do your homework or did poorly on a test. We feared him, trembled when he reprimanded us, and were scared that he would contact our parents. Yet we revered him, and he became a role model to many of us. Above all, he showed us the relevance of mathematics to the real world - especially to physics. And that meant learning a good deal of trigonometry.

He and I have kept a lively correspondence for many years, and we have met several times. He was very opinionated, and whatever you said about any subject - mathematical or otherwise - he would argue with you, and usually prevail. Years after I finished my university studies, he would let me understand that he was still my teacher. Born in China to a family that fled Europe before World War 11, he emigrated to Israel and began his education at the Hebrew University of Jerusalem, only to be drafted into the army during Israel's war of independence. Later he joined the faculty of Tel Aviv University and was granted tenure despite not having a Ph.D. - one of only two faculty members so honored. In 1989, while giving his weekly lecture on the history of mathematics, he suddenly collapsed and died instantly. His name was Nathan Elioseph. I miss him dearly.

And now the frustration. In the late 1950s, following the early Soviet successes in space (Sputnik I was launched on October 4, 1957; 1 remember the date-it was my twentieth birthday) there was a call for revamping our entire educational system, especially science education. New ideas and new programs suddenly proliferated, all designed to close the perceived technological gap between us and the Soviets (some dared to question whether the gap really existed, but their voices were swept aside in the general frenzy). These were the golden years of American science education. If you had some novel idea about how to teach a subject-and often you didn't even need that muchyou were almost guaranteed a grant to work on it. Thus was born the "New Math" - an attempt to make students understand what they were doing, rather than subject them to rote learning and memorization, as had been done for generations. An enormous amount of time and money was spent on developing new ways of teaching math, with emphasis on abstract concepts such as set theory, functions (defined as sets of ordered pairs), and formal logic. Seminars, workshops, new curricula, and new texts were organized in haste, with hundreds of educators disseminating the new ideas to thousands of bewildered teachers and parents. Others traveled abroad to spread the new gospel in developing countries whose populations could barely read and write.

Today, from a distance of four decades, most educators agree that the New Math did more harm than good. Our students may have been taught the language and symbols of set theory, but when it comes to the simplest numerical calculations they stumble-with or without a calculator. Consequently, many high school graduates are lacking basic algebraic skills, and, not surprisingly, some 50 percent of them fail their first college- level calculus course. Colleges and universities are spending vast resources on remedial programs (usually made more palatable by giving them some euphemistic title like "developmental program" or "math lab"), with success rates that are moderate at best.

Two of the casualties of the New Math were geometry and trigonometry. A subject of crucial importance in science and engineering, trigonometry fell victim to the call for change. Formal definitions and legalistic verbosity-all in the name of mathematical rigor-replaced a real understanding of the subject. Instead of an angle, one now talks of the measure of an angle; instead of defining the sine and cosine in a geometric context as ratios of sides in a triangle or as projections of the unit circle on the x- and y-axes - one talks about the wrapping function from the reals to the interval [-1, 1]. Set notation and set language have pervaded all discussion, with the result that a relatively simple subject became obscured in meaningless formalism.

Worse, because so many high school graduates are lacking basic algebraic skills, the level and depth of the typical trigonometry textbook have steadily declined. Examples and exercises are often of the simplest and most routine kind, requiring hardly anything more than the memorization of a few basic formulas. Like the notorious "word problems" of algebra, most of these exercises are dull and uninspiring, leaving the student with a feeling of "so what?" Hardly ever are students given a chance to cope with a really challenging identity, one that might leave them with a sense of accomplishment. For example,

1. Prove that for any number x,

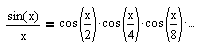
This formula was discovered by Euler. Substituting x = u12, using the fact that cos 7T/4 = /2-/2 and repeatedly applying the half-angle formula for the cosine, we get the beautiful formula

discovered in 1593 by François Viète in a purely geometric way.

2. Prove that in any triangle,

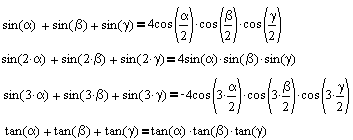
(The last formula has some unexpected consequences, which we will discuss in chapter 12.) These formulas are remarkable for their symmetry; one might even call them "beautiful" - a kind word for a subject that has undeservedly gained a reputation of being dry and technical. In Appendix 3, 1 have collected some additional beautiful formulas, recognizing of course that "beauty" is an entirely subjective trait.

"Some students," said Edna Kramer in The Nature and Growth of Modem Mathematics, consider trigonometry "a glorified geometry with superimposed computational torture." The present book is an attempt to dispel this view. I have adopted a historical approach, partly because I believe it can go a long way to endear mathematics-and science in general-to the students. However, I have avoided a strict chronological presentation of topics, selecting them instead for their aesthetic appeal or their relevance to other sciences. Naturally, my choice of subjects reflects my own preferences; numerous other topics could have been selected.

The first nine chapters require only basic algebra and trigonometry; the remaining chapters rely on some knowledge of calculus (no higher than Calculus 11). Much of the material should thus be accessible to high school and college students. Having this audience in mind, I limited the discussion to plane trigonometry, avoiding spherical trigonometry altogether (although historically it was the latter that dominated the subject at first). Some additional historical material-often biographical in nature-is included in eight "sidebars" that can be read independently of the main chapters. If even a few readers will be inspired by these chapters, I will consider myself rewarded.

Skokie, Illinois
February 20, 1997

|Up|

|Contact| |Front page| |Contents| |Books|

Copyright © 1996-2018 Alexander Bogomolny

71544166