Two Equations in Three Variables

Dan Sitaru has kindly posted a problem (see below) at the CutTheKnotMath facebook page. Solution 1 is by Daniel Liu; Solution 2 is by Leo Giugiuc and Dan Sitaru; Solution 3 is by Tran Van Hien.

All three solutions below use the substitution $a=e^x,\;$ $b=e^y\;,$ $c=e^z$ to reduce the system to

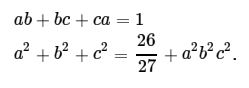
where $a,b,c\gt 0.$

By the rearrangement inequality, $a^2+b^2+c^2\ge ab+bc+ca=1,\;$ and, by the AM-GM inequality $1=ab+bc+ca\ge 3\sqrt[3]{a^2b^2c^2},\;$ implying $\displaystyle\frac{1}{27}\ge a^2b^2c^2.$

Thus $\displaystyle a^2+b^2+c^2\ge\frac{26}{27}+a^2b^2c^2$ with equality only if $\displaystyle a=b=c=\frac{1}{\sqrt{3}}.\;$ This gives a solution to the original problem:

$\displaystyle x=y=z=-\frac{1}{2}\ln 3.$

Since $a^2+b^2+c^2\ge ab+bc+ca,\;$ then $a^2+b^2+c^2\ge 1,\;$ implying $\displaystyle a^2b^2c^2\ge\frac{1}{27},\;$ i.e., $\displaystyle (a^2b^2c^2)^{\frac{2}{3}}\ge\frac{1}{3}.\;$

By the AM-GM inequality, $\displaystyle\frac{ab+bc+ca}{3}\ge (abc)^{\frac{2}{3}},\;$ so that $\displaystyle (abc)^{\frac{2}{3}}\le\frac{1}{3}.$ Thus we have $\displaystyle (abc)^{\frac{2}{3}}=\frac{1}{3}.$

This means that $\displaystyle\frac{ab+bc+ca}{3}=(abc)^{\frac{2}{3}},\;$ implying $\displaystyle ab=bc=ca=\frac{1}{3}\;$ and, subsequently, $\displaystyle a=b=c=\frac{1}{\sqrt{3}}.\;$ Thus

$\displaystyle x=y=z=-\frac{1}{2}\ln 3.$

On one hand, we have

$\displaystyle 1 = e^{x+y}+y^{y+z}+e^{z+x} \ge 3\sqrt[3]{e^{2x+2y+2z}},$

implying $\displaystyle e^{2x+2y+2z}\le\frac{1}{27}.$

On the other hand, by the Rearrangement inequality,

$1 = e^{x+y}+y^{y+z}+e^{z+x}= 1 = e^x\cdot e^y+y^y\cdot e^z+e^z\cdot e^x\le e^{2x}+e^{2y}+e^{2z}.$

Combining the wo gives

$\displaystyle 1\le e^{2x}+e^{2y}+e^{2z}=\frac{26}{27}+e^{2x+2y+2z}\le 1,$

implying $\displaystyle x=y=z=\ln\frac{1}{\sqrt{3}}-\frac{\ln 3}{2}.$

Multiplying successively $ab+bc+ca=1\;$ by $a,b,c\;$ gives

$a^2(b+c)=1-abc,\\ b^2(c+a)=1-abc,\\ c^2(a+b)=1-abc.$

Thus, e.g., $a^2(b+c)=b^2(c+a),\;$ or $ab(a-b)=c(b^2-a^2).\;$ Necessarily, $a=b\;$ because, otherwise, $ab=-c(a+b)\;$ in contradiction with $a,b,c\gt 0.$ Similarly, $b=c.\;$ Thus the only possibility is $\displaystyle a=b=c=\frac{1}{\sqrt{3}}\;$ and, subsequently,

$\displaystyle x=y=z=-\frac{1}{2}\ln 3.$