Dan Sitaru's Cyclic Inequality In One Variable
Problem
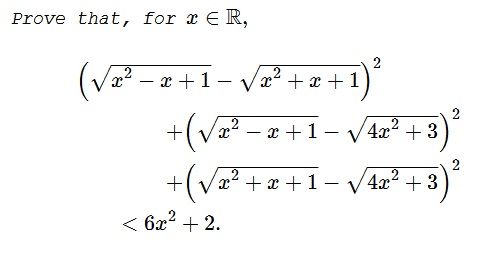
Solution 1
For typographic convenience, let's denote $a=x^2-x+1,\,$ $b=x^2-x+1,\,$ and $c=4x^2+3.\,$ Then, upon squaring the required inequality takes an equivalent form:
$6x^2+8 = 12x^2+10-(6x^2+2) \lt 2(\sqrt{ab}+\sqrt{bc}+\sqrt{ca}),$
or,
$3x^2+4 \lt \sqrt{ab}+\sqrt{bc}+\sqrt{ca}.$
Squaring once more gives
$(3x^2+4)^2-(ab+bc+ca)\lt 2(a\sqrt{bc}+b\sqrt{ca}+c\sqrt{ab}),$
which reduces to
(*)
$9(x^2+1)\lt 2(a\sqrt{bc}+b\sqrt{ca}+c\sqrt{ab}).$
Now,
$\displaystyle\begin{align} a&=x^2-x+1=\left(x-\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4},\\ b&=x^2+x+1=\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4},\\ c&=4x^2+3\ge 3. \end{align}$
The right-hand side of (*) is then estimated as
$\displaystyle\begin{align} RHS(*) &\gt 2(a\frac{3}{2}+b\frac{3}{2}+c\frac{3}{4})\\ &=3(2x^2+2)+\frac{3}{2}(4x^2+3)\\ &=12x^2+\frac{21}{2}\\ &\gt 9(x^2+1)=LHS(*). \end{align}$
Solution 2
Let's denote $b=\sqrt{x^2-x+1},\,$ $c=\sqrt{x^2-x+1},\,$ and $a=\sqrt{4x^2+3}.\,$ Note that $a,b,c\,$ are the sides of a triangle: $a+b\gt c,\,$ b+c\gt a,\,$ $c+a\gt b.\,$
For example, square $b+c\gt a\,$ to obtain $2(x^2+1)+2\sqrt{(x^2+1)^2-x^2}\gt 4x^2+3,\,$ or $2\sqrt{(x^2+1)^2-x^2}\gt2x^2+1;\,$ squaring the second time: $4x^4+4x^2+4\gt 4x^4+4x^2+1,\,$ which is true.In $\Delta ABC,$
$\displaystyle\begin{align} \cos A &= \frac{b^2+c^2-a^2}{2bc}=\frac{-2x^2-1}{2\sqrt{x^4+x^2+1}},\\ \sin A &= \sqrt{1-\left(\frac{-2x^2-1}{2\sqrt{x^4+x^2+1}}\right)^2}\\ &=\sqrt{\frac{3}{4(x^4+x^2+1)}},\\ S &=\frac{1}{2}bc\sin A=\frac{1}{2}\cdot\sqrt{x^4+x^2+1}\cdot\sqrt{\frac{3}{4(x^4+x^2+1)}}\\ &=\frac{\sqrt{3}}{4}. \end{align}$.
By the Hadwiger-Finsley inequality, $\displaystyle\sum_{cycl}(a-b)^2+4S\sqrt{3}\lt\sum_{cycl}a^2\,$ such that
$\displaystyle\sum_{cycl}(a-b)^2+4\sqrt{3}\cdot\frac{\sqrt{3}}{4}\lt x^2-x+1+x^2+x+1+4x^2+3,$
i.e., $\displaystyle\sum_{cycl}(a-b)^2\lt 6x^2+2.\,$ This is exactly the required inequality.
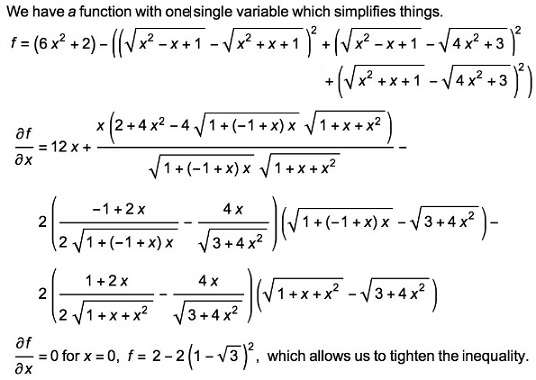
Solution 3
Acknowledgment
Dan Sitaru has kindly posted a problem from the Romanian Mathematical Magazine, with a solution (Solution 1) by Soumava Chakraborty. Solution 2 is by Dan Sitaru; Solution 3 is by Nassim N. Taleb.
|Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73295240
