Circle, Isosceles Triangle and Fixed Point
What is this about?
A Mathematical Droodle

---

This applet requires Sun's Java VM 2 which your browser may perceive as a popup. Which it is not. If you want to see the applet work, visit Sun's website at http://www.java.com/en/download/index.jsp, download and install Java VM and enjoy the applet.

---

Explanation

|Activities| |Contact| |Front page| |Contents| |Geometry| |Store|

The applet may suggest the following a problem from the 2006 Irish MO:

P and Q are points on the equal sides AB and AC respectively of an isosceles triangle ABC such that AP = CQ. Moreover, neither P nor Q is a vertex of ABC. Prove that the circumcircle of the triangle APQ passes through the circumcenter of the triangle ABC.

---

This applet requires Sun's Java VM 2 which your browser may perceive as a popup. Which it is not. If you want to see the applet work, visit Sun's website at http://www.java.com/en/download/index.jsp, download and install Java VM and enjoy the applet.

---

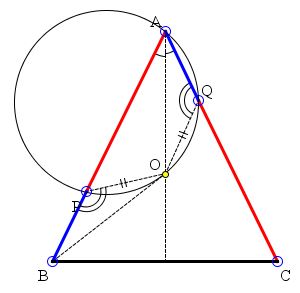
Let C(APQ) be the circumcircle of Δ. Let O be the second intersection of C(APQ) with the altitude from the apex A. Consider two triangles: BPO and AQO. The idea is to prove that the triangles are congruent, from which it would follow that AO = BO. Since AO is the axis of summetry of the isosceles triangle ABC, BO = CO, implying that the three distances from O to the vertices of ΔABC are equal and making O the circumcenter.

For details see a wiki write-up. There are also extra five solutions.

|Activities| |Contact| |Front page| |Contents| |Geometry| |Store|