Pythagorean Theorem
A Family of Proofs
More than a hundred years ago The American Mathematical Monthly published a series of short notes listing great many proofs of the Pythagorean theorem. The authors, B. F. Yanney and J. A. Calderhead, went an extra mile counting and classifying proofs of various flavors. Proof V from their collection (Am Math Monthly, v.3, n. 4 (1896), 110-113. This is #56 on our page) provides a sample of their thoroughness. Based on the diagram below they counted as many as 4864 different proofs. Let's follow in their footsteps and see how this came about.
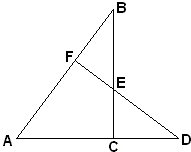
Triangle ABC is right angled at C, F lies on AB, D on, say, AC extended beyond C, DF perpendicular to AB. Let E be the intersection of DF and BC. There are thus four similar right triangles.
Letting, as usual,
| (1) | b:z :: c:b+x | b(b + x) = cz | |
| (2) | b:z :: a:v+w | b(v + w) = az | |
| (3) | c:b+x :: a:v+w | c(v + w) = a(b + x) | |
| (4) | b:y :: c:w | bw = cy | |
| (5) | b:y :: a:x | bx = ay | |
| (6) | c:w :: a:x | cx = aw | |
| (7) | b:v :: c:a-y | b(a - y) = cv | |
| (8) | b:v :: a:c-z | b(c - z) = av | |
| (9) | c:a-y :: a:c-z | c(c - z) = a(a - y) | |
| (10) | z:y :: b+x:w | zw = y(b + x) | |
| (11) | z:y :: v+w:x | xz = y(v +w) | |
| (12) | b+x:w :: v+w:x | x(b + x) = w(v +w) | |
| (13) | z:v :: b+x:a-y | z(a - y) = v(b + x) | |
| (14) | z:v :: v+w:c-z | z(c - z) = v(v + w) | |
| (15) | b+x:a-y :: v+w:c-z | (c - z)(b + x) = (a - y)(v + w) | |
| (16) | y:v :: w:a-y | y(a - y) = vw | |
| (17) | y:v :: x:c-z | y(c - z) = vx | |
| (18) | w:a-y :: x:c-z | w(c - z) = x(a - y) |
We are now to find combinations of the above equations from which elements x, y, z, v, w can be eliminated, thus leaving us the relation existing between a, b and c.
It is evident that from no single equation, nor from any set of two equations, can the relation be determined.
There remain three possible cases of combinations to be considered:
- When three of the elements x, y, z, v, w are involved.
- When four.
- When five, or all.
First case. In this case there are
Before taking up this in detail, we note that by inspection of proportions, it easily may be seen that the following eighteen sets of equations each comprise dependent equations:
1, 2, 3; 4, 5, 6; 7, 8, 9;
Hence, in our search for possible combinations, all such must be rejected as contain any of these sets.
There are three equations involving v, w, x: 3, 6, 12. But this combination must be rejected for the reason just given. For the same reason, or because there is wanting a sufficient number of equations involving the three unknown elements, the other nine combinations must be rejected, except the combination x, y, z, which elements are involved in equations 1, 5, 9. If we eliminate x, y, z from these equations, we obtain the desired relation,
It should be observed, in passing, that future combinations including 1, 5, 9, must also be rejected.
Second case. Of this case there are
Before proceeding to investigate this case, it is necessary to call attention to the sets of four dependent equations. Take, for example, the set
The equations involving the unknown elements
Similarly, we find twenty one sets each of four equations, involving
Third case. Of this case, there are
To determine how many of this number must be rejected, proceed as follows. Begin with the list of the sets of dependant equations found in Case 1. Notice that there are
One more class must be rejected: sets of five dependent equations. For example, 10, 9, 7, 6, 3 which is a type of all the others - 72 in number - and from which the 72 can easily be deduced.
Deducting from 8568, 3746 + 73, we have remaining 4749 sets of five, from which can be derived the identity
Therefore, 1 + 114 + 4749 = 4864, the number of proofs by this method.
Examples 1:
| c(v + w) = a(b + x) | (3) | |
| bw = cy | (4) | |
| bw = cy | (6) | |
| b(a - y) = cv | (7) | |
| bx = ay | (5); also (4) in (6) | |
| ab - by + c²y/b - a²y/b = ab | (7, 4, 5) in (3). | |
| c²/b - a²/b = b | etc. |
Examples 2:
| b(b + x) = cz | (1) | |
| b(v + w) = az | (2) | |
| bw = cy | (4) | |
| bx = ay | (5) | |
| b(a - y) = cv | (7) | |
| cv + cw - ax = ab | (3); also (1) in (2) | |
| ab - by + c²y/b - a²y/b = ab | (7, 4, 5) in (3) | |
| c²/b - a²/b = b | etc. |
![]()
|Pythagorean Theorem| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny71546189
