An Identity with Inradius and Circumradii
Problem
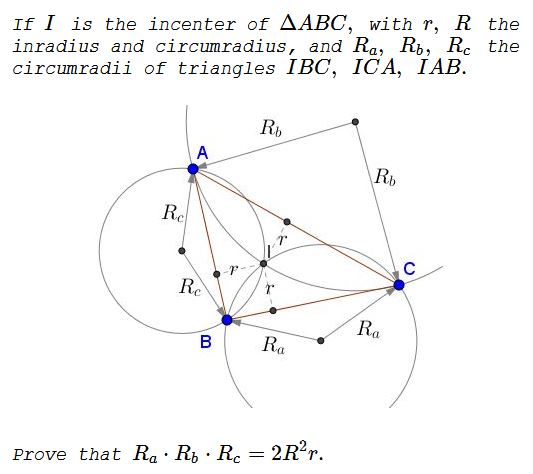
Solution 1
In every triangle with side lengths $a,b,c,\,$ area $S\,$ and circumradius $R,\,$ $abc=4RS.\,$ So, in $\Delta IAB,\,$
$\displaystyle R_c=\frac{AI\cdot BI\cdot AB}{4[\Delta IAB]}=\frac{\displaystyle \frac{r}{\sin\frac{A}{2}}\cdot\frac{r}{\sin\frac{B}{2}}\cdot c}{\displaystyle 4\frac{rc}{2}}=\frac{r}{\displaystyle 2\sin\frac{A}{2}\cdot\sin\frac{B}{2}},$
etc. So that
$\displaystyle \begin{align} \prod_{cycl}R_a &= \frac{r^3}{\displaystyle 8\prod_{cycl}\sin^2\frac{A}{2}}=\frac{r^3}{\displaystyle 8\prod_{cycl}\frac{(s-b)(s-c)}{bc}}\\ &=\frac{r^3a^2b^2c^2s^2}{8S^4}=\frac{16S^2r^3s^2}{8S^4}=\frac{16r^3s^2}{8S^2}\\ &=2R^2r. \end{align}$
Solution 2
$\displaystyle\begin{align} BC&=2R_a\sin\angle BIC=2R_a\sin\left(\pi-\frac{B+C}{2}\right)\\ &=2R_a\sin\frac{B+C}{2}.\, \end{align}$
On the other hand,
$\displaystyle\begin{align} BC&=2R\sin A=2R\sin (A+B)\\ &=4R\sin\frac{B+C}{2}\cos\frac{B+C}{2}.\, \end{align}$
It follows that $\displaystyle R_a=2R\sin\frac{A}{2},\,$ etc. We conclude that
$\displaystyle R_a\cdot R_b\cdot R_c=8R^3\sin\frac{A}{2}\cdot\sin\frac{B}{2}\cdot\sin\frac{C}{2}=2R^2r.$
Solution 3
If $O_a\,$ is the circumcenter of $\Delta IBC\,$ then $O_aB=O_aI=O_aC=R_a.\,$
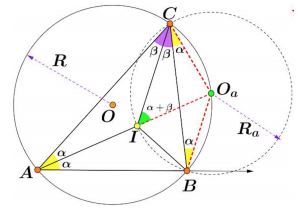
In $\Delta AO_aB,\,$ $O_aB=2R\sin\alpha,\,$ implying $R_a=2R\sin\displaystyle\frac{A}{2}.\,$ Similarly, $R_b=2R\sin\displaystyle\frac{B}{2}\,$ and $R_c=2R\sin\displaystyle\frac{C}{2}.\,$
$\displaystyle R_a\cdot R_b\cdot R_c=2R^2\left(4R\sin\frac{A}{2}\cdot\sin\frac{B}{2}\cdot\sin\frac{C}{2}\right)=2R^2r.$
Hence, $R_a\cdot R_b\cdot R_c=2R^2r.$
Acknowledgment
This problem by Mehmet Şahin (Turkey) has been posted to the site of the Romanian Mathematical Magazine and communicated to me by Dan Sitaru. Solution 1 is by Dan Sitaru (Romania); Solution 2 is by Marian Dincă (Romania); Solution 3 is by Miguel Ochoa Sanchez (Peru).
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny71536740
