A proof of the butterfly theorem using the scale factor between the two wings.
Martin Celli
October 21st 2016
Theorem (The Butterfly Theorem)
Let $M\;$ be the midpoint of a chord $PQ\;$ of a circle, through which two other chords $AB\;$ and $CD\;$ are drawn. Let us assume that $A\;$ and $D\;$ do not belong to a same half-plane defined by $PQ.\;$ Let $X\;$ (respectively $Y)\;$ be the intersection of $AD\;$ (respectively $BC)\;$ and $PQ.$
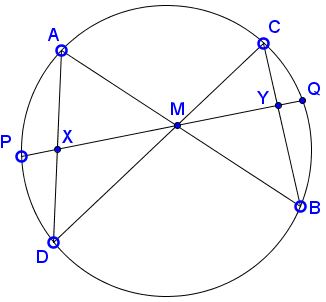
Then $M\;$ is also the midpoint of $XY.$
Let $O\;$ be the center of the circle. The points $A\;$ and $C\;$ belong to a same half-plane defined by $PQ,\;$ the points $B\;$ and $D\;$ to the other half-plane. For sake of simplicity, we can assume that $O\;$ belongs to the same half-plane as $B\;$ and $D.$
In the proof, we directly show that the ratio $\displaystyle\frac{\sin(CYM)}{\sin(AXM)}\;$ is nothing but the scale factor between the two wings $AMD\;$ and $CMB,\;$ which are similar by the Inscribed Angle Theorem:
$\displaystyle\frac{\sin(CYM)}{\sin(AXM)}=\alpha,\;$ where $\displaystyle\alpha=\frac{CM}{AM}=\frac{BM}{DM}=\frac{CB}{AD}.$
More precisely, we have:
$\displaystyle\sin(AXM)=\frac{DM^2-AM^2}{AD\cdot OM}.$
As a matter of fact:
$\displaystyle\begin{align} DM^2-AM^2 &= ||\overrightarrow{DO}+\overrightarrow{OM}||^2-||\overrightarrow{AO}+\overrightarrow{OM}||^2\\ &=OD^2+OM^2+2\overrightarrow{DO}\cdot\overrightarrow{OM}-(OA^2+OM^2+2\overrightarrow{AO}\cdot\overrightarrow{OM})\\ &=2\overrightarrow{DO}\cdot\overrightarrow{OM}\; \text{since }OA=OD\\ &=2AD\cdot OM\sin(AXM)\;\text{as } OMX=OMY=\frac{MX+OMY}{2}=\frac{\pi}{2}, \end{align}$
because triangle $OMP\;$ and $OMQ\;$ are congruent. Similarly, we have:
$\displaystyle\sin(CYM)=\frac{BM^2-CM^2}{CB\cdot OM}.$
Thus:
$\displaystyle\begin{align} \frac{XM}{YM}&=\frac{AM}{CM}\cdot\frac{CM}{YM}\cdot\frac{XM}{AM}\\ &=\frac{AM}{CM}\cdot\frac{\sin(CYM)}{\sin(YCM)}\cdot\frac{\sin(XAM)}{\sin(AXM)} \end{align}$
by the law of sines, applied in triangles $AXM\;$ and $CYM.\;$ Now we continue using the Inscribed Angle Theorem, as the next step:
$\displaystyle\begin{align} \frac{XM}{YM}&=\frac{AM}{CM}\cdot\frac{CM}{YM}\cdot\frac{XM}{AM}\\ &=\frac{AM}{CM}\cdot\frac{\sin(CYM)}{\sin(AXM)}\\ &=\frac{AM}{CM}\cdot\frac{BM^-CM^2}{CB\cdot OM}\cdot\frac{AD\cdot OM}{DM^2-AM^2}\\ &=\frac{AM}{CM}\cdot\frac{AD}{CB}\cdot\frac{BM^2-CM^2}{DM^2-AM2}\\ &=\frac{1}{\alpha}\cdot\frac{1}{\alpha}\cdot\alpha^2\\ &=1. \end{align}$
Butterfly Theorem and Variants
- Butterfly theorem
- 2N-Wing Butterfly Theorem
- Better Butterfly Theorem
- Butterflies in Ellipse
- Butterflies in Hyperbola
- Butterflies in Quadrilaterals and Elsewhere
- Pinning Butterfly on Radical Axes
- Shearing Butterflies in Quadrilaterals
- The Plain Butterfly Theorem
- Two Butterflies Theorem
- Two Butterflies Theorem II
- Two Butterflies Theorem III
- Algebraic proof of the theorem of butterflies in quadrilaterals
- William Wallace's Proof of the Butterfly Theorem
- Butterfly theorem, a Projective Proof
- Areal Butterflies
- Butterflies in Similar Co-axial Conics
- Butterfly Trigonometry
- Butterfly in Kite
- Butterfly with Menelaus
- William Wallace's 1803 Statement of the Butterfly Theorem
- Butterfly in Inscriptible Quadrilateral
- Camouflaged Butterfly
- General Butterfly in Pictures
- Butterfly via Ceva
- Butterfly via the Scale Factor of the Wings
- Butterfly by Midline
- Stathis Koutras' Butterfly
- The Lepidoptera of the Circles
- The Lepidoptera of the Quadrilateral
- The Lepidoptera of the Quadrilateral II
- The Lepidoptera of the Triangle
- Two Butterflies Theorem as a Porism of Cyclic Quadrilaterals
- Two Butterfly Theorems by Sidney Kung
- Butterfly in Complex Numbers
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny
71536847
