Kiepert's Centroid
What is this about?
Problem
On the side of $\Delta ABC$ construct similarly oriented isosceles (Kiepert) triangles $ABN_{c},$ $BCN_a,$ and $CAN_b.$
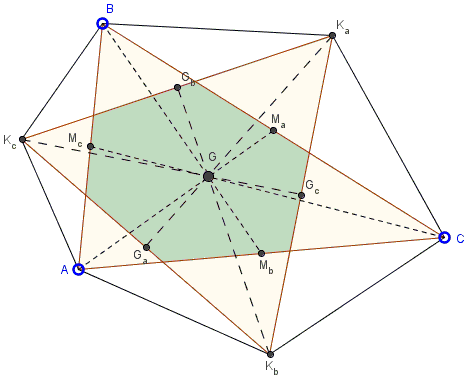
Prove that the centroids of triangles $ABC$ and $N_{a}N_{b}N_{c}$ coincide.
Hint
I think in complex numbers the proof is exceptionally simple. In affine geometry, though, it is no more difficult.
Solution
Let in a complex plain, the vertices of $\Delta ABC$ correspond to complex numbers $\alpha,$ $\beta,$ $\gamma.$

Then, if $i^{2}=-1$,
$N_{a}=(\beta +\gamma)/2 + t(\beta -\gamma)i,$
$N_{b}=(\gamma + \alpha)/2 + t(\gamma -\alpha)i,$
$N_{c}=(\alpha +\beta)/2 + t(\alpha -\beta)i,$
for some real $t$ that determines the base angles of the isosceles triangles.
The centroid of $\Delta ABC$ is given by $(\alpha +\beta +\gamma)/3$ while that of $\Delta N_{a}N_{b}N_{c}$ is
$\displaystyle \begin{align} \frac{(\beta +\gamma)/2 + t(\beta -\gamma)i}{3} &+ \frac{(\gamma + \alpha)/2 + t(\gamma -\alpha)i}{3} \\ &+ \frac{(\alpha +\beta)/2 + t(\alpha -\beta)i}{3} \\ &= \frac{\alpha +\beta +\gamma}{3}. \end{align} $
Acknowledgment
I am not sure where this problem comes from. I've been cleaning my folder of GeoGebra projects when I noticed this one. I'd be grateful if somebody steps forward.
Grégoire Nicollier has kindly pointed out in private correspondence that the above has an immediate extension to similar triangles of arbitrary shape. I placed that in a separate page.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny71532585
