An Inequality with Cubic Roots
Problem
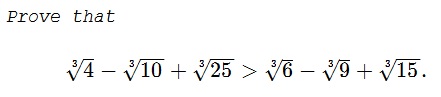
Solution 1
Rewrite the inequality as
$\displaystyle \sqrt[3]{4}+\sqrt[3]{9}+\sqrt[3]{25}\gt\sqrt[3]{6}+\sqrt[3]{10}+\sqrt[3]{15}.$
If $a=\sqrt[3]{2},$ $b=\sqrt[3]{3},$ $c=\sqrt[3]{5}$ then the inequality to prove becomes
$a^2+b^2+c^2\gt ab+bc+ca.$
We have several proofs for $a^2+b^2+c^2\ge ab+bc+ca,$ with the equality when $a=b=c.$
Solution 2
For $n\ge 2,$ set $a=\sqrt[n]{5},$ $b=\sqrt[n]{3},$ $c=\sqrt[n]{2}.$ Then the inequality
$\displaystyle \sqrt[n]{4}+\sqrt[n]{9}+\sqrt[n]{25}\gt\sqrt[n]{6}+\sqrt[n]{10}+\sqrt[n]{15}$
can be rewritten as $a^2+b^2+c^2\gt ab+bc+ca,$ with a solution as above.
Solution 3
Set
$A=\sqrt[3]{4}-\sqrt[3]{10}+\sqrt[3]{25}=2^{\frac{2}{3}}+5^{\frac{1}{3}}\left(5^{\frac{1}{3}}-2^{\frac{1}{3}}\right),\\ B=\sqrt[3]{6}-\sqrt[3]{9}+\sqrt[3]{15}=2^{\frac{1}{3}}+3^{\frac{1}{3}}+3^{\frac{1}{3}}\left(5^{\frac{1}{3}}-2^{\frac{1}{3}}\right).$
Then
$\displaystyle \begin{align} A-B\;&=-2^{\frac{1}{3}}\left(3^{\frac{1}{3}}-2^{\frac{1}{3}}\right)+5^{\frac{1}{3}}\left(5^{\frac{1}{3}}-2^{\frac{1}{3}}\right)-3^{\frac{1}{3}}\left(5^{\frac{1}{3}}-3^{\frac{1}{3}}\right)\\ &\,\gt -2^{\frac{1}{3}}\left(3^{\frac{1}{3}}-2^{\frac{1}{3}}\right)+3^{\frac{1}{3}}\left(5^{\frac{1}{3}}-2^{\frac{1}{3}}\right)-3^{\frac{1}{3}}\left(5^{\frac{1}{3}}-3^{\frac{1}{3}}\right)\\ &=-2^{\frac{1}{3}}\left(3^{\frac{1}{3}}-2^{\frac{1}{3}}\right)+3^{\frac{1}{3}}\left(3^{\frac{1}{3}}-2^{\frac{1}{3}}\right)\\ &=\left(3^{\frac{1}{3}}-2^{\frac{1}{3}}\right)^2\gt 0. \end{align}$
Acknowledgment
This is a problem from R. Honsberger's Mathematical Chestnuts from Around the World (MAA, 2001, p 136.) The problem was originally offered at the 1984 Leningrad Mathematical Olympiad.
Solution 2 is by Michel Charbonneau; Solution 3 is by Daniele Pinna.
![]()
|Contact| |Up| |Front page| |Contents| |Arithmetic|
Copyright © 1996-2018 Alexander Bogomolny71536530
