A Case for Determinants
Problem
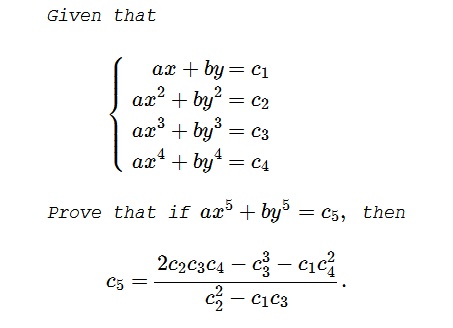
Solution
Denote $x+y=s\,$ and $xy=p.\,$ Then
$\begin{align} c_2s&=(ax^2+by^2)(x+y)=ax^3+by^3+xy(ax+by)\\ &=c_3+c_1p \end{align}$
Similarly, $c_3s=c_4+c_2p\,$ and $c_4s=c_5+c_3p.\,$ Thus, the linear system
$\left(\begin{array}{ccc}-c_2 & c_3 & c_1\\-c_3 & c_4 & c_2\\-c_4 & c_5 & c_3\end{array}\right)\left(\begin{array}{c}\,s\\1\\p\end{array}\right)=\mathbf{0}$
has a non-trivial solution, implying that the determinant of the matrix equals zero:
$D=\left|\begin{array}{ccc}-c_2 & c_3 & c_1\\-c_3 & c_4 & c_2\\-c_4 & c_5 & c_3\end{array}\right|=-\left|\begin{array}{ccc}c_2 & c_3 & c_1\\c_3 & c_4 & c_2\\c_4 & c_5 & c_3\end{array}\right|=0.$
Expanding by the last row we obtain
$c_4\left|\begin{array}{cc}\,c_3 & c_1\\c_4 & c_2\end{array}\right|+c_3\left|\begin{array}{cc}\,c_2 & c_3\\c_3 & c_4\end{array}\right|=c_5\left|\begin{array}{cc}\,c_2 & c_1\\c_3 & c_2\end{array}\right|.$
Further expansion of the $2\times 2\,$ determinant proves the statement and at the same time unveils the mystery of the identity
$\displaystyle c_5=\frac{2c_2c_3c_4-c_3^2-c_1c_4^2}{c_2^2-c_1c_3}.$
Acknowledgment
The problem has been kindly posted at the CutTheKnotMath facebook page by Leo Giugiuc with a link to a post by Francisco Javier Gracía Capitán who had referred to Problem 15 at AIME 1990. His is a generalization of the latter.
![]()
|Contact| |Up| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny71529912
