An Inequality from Gazeta Matematica, March 2016 III

Several inequalities with solution by Dan Sitaru and Leo Giugiuc have been just published in Gazeta Matematica (March 2016). Here is one with two of its applications and a proof (Proof 1)from the article. Along the way several additional proofs have been added. Proof 2 is by Imad Zak; Proof 3 is by Emil Stoyanov; Proof 4 is by Grégoire Nicollier; Proof 5 is by Kunihiko Chikaya; Proofs 6 and 7 are by Robert Kosova; Proof 8 by Abdur Rahman.

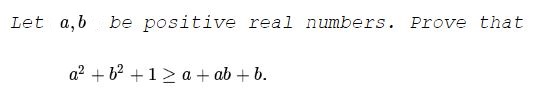
(Marco Antônio Manetta has observed that the requirement that the three numbers are positive is redundant.)

Define $A=\left(\begin{array}\;1&a&b\\a&b&1\end{array}\right).$ By the Binet-Cauchy theorem, $\det(AA^T)\ge 0.\;$ But

$\det(AA^T)=(a^2+b^2+1)^2-(a+ab+b)^2,$

proving the inequality at hand.

Let $S=a+b\;$ and $P=ab,\;$ by the AM-GM inequality, we have $\displaystyle P\le \frac{S^2}{4}\;$ and the required inequality is equivalent to $S^2-S+1\ge 3P,\;$ so suffice it to prove that $\displaystyle S^2-S+1\ge\frac{3S^2}{4}\;$ which is equivalent to $\displaystyle\frac{S^2}{4}-S+1\ge 0,\;$ or $\displaystyle (\frac{S}{2}-1)^2\ge 0\;$ which is clearly true. The equality holds when $S=2\;$ and $P=1,\;$ i.e., when $a=b=1.$

The required inequality is equivalent to $a^2-(b+1)a+(b^2-b+1)\ge 0.\;$ Consider the quadratic function $f(x)=x^2-(b+1)x+(b^2-b+1)\ge 0.\;$ Its discriminant $D=(b+1)^2-4b^2+4b-4=-3(b-1)^2\;$ is never positive, implying that function $f\;$ is never negative.

The inequality reduces to to $(a-1)^2 + (b-1)^2 \ge (a-1)(b-1)\;$ which could be strengthened to $(a-1)^2 + (b-1)^2 \ge 2(a-1)(b-1).$

The required inequality is equivalent to

$(a+b-2)^2+3(a-b)^2\ge 0.$

The inequality is equivalent to $2a^2+2b^2+2\ge 2a+2b+2ab.$

But $2a\le a^2+1,\;$ $2b\le b^2+1.\;$ Thus suffice it to show that

$2a^2+2b^2+2\ge (a^2+1)+(b^2+1) +2ab,$

or, $a^2+b^2\ge 2ab,\;$ which is $(a-b)^2\ge 0,\;$ and is true.

$\begin{align} a^2+b^2 &\ge 2ab\\ a^2+1 &\ge 2a\\ b^2+1 &\ge 2b. \end{align}$

Adding up gives $2a^2+2b^2+2\ge 2a+2ab+2b,\;$ and we only have to divide by $2.$

By the Cauchy-Schwarz inequality,

$\begin{align} (a+ab+b)^2 &= (a\cdot 1+ b\cdot a+1\cdot b)\\ &\le (a^2+b^2+1)(1+a^2+b^2), \end{align}$

implying $a+ab+b\le a^2+b^2+1.$

By the AM-QM inequality,

$\displaystyle a^2+b^2+1\ge \frac{1}{2}(a+b)^2+1.$

Suffice it to prove that

$(a+b)^2+2\ge 2a+2ab+2b.$

But this is equivalent to $(a-1)^2+(b-1)^2\ge 0,\;$ which is obvious.

$\displaystyle\prod_{1\le i\le j\le n}(i^2+j^2+1)\ge n!\prod_{1\le i\le j\le n}(2+\sqrt{ij}).$

Observe that $a^2+b^2+1\ge a+b+ab\ge ab+2\sqrt{ab}=\sqrt{ab}(2+\sqrt{ab}).\;$ Using this,

$\displaystyle\begin{align} \prod_{1\le i\le j\le n}(i^2+j^2+1) &\ge \prod_{1\le i\le j\le n}\sqrt{ij}(2+\sqrt{ij})\\ &=\prod_{1\le i\le j\le n}\sqrt{ij}\prod_{1\le i\le j\le n}(2+\sqrt{ij})\\ &=n!\prod_{1\le i\le j\le n}(2+\sqrt{ij}). \end{align}$

Obviously, the inequality can be strengthened.

Show that $\displaystyle\int_{0}^{\frac{\pi}{2}}\frac{1}{\sin x+\sin x\cos x+\cos x}dx\ge\frac{\pi}{4}.$

Set $a=\sin x\;$ and $b=\cos x.\;$ Then $2\ge 1+\sin^2x+\cos^2x\ge \sin x+\sin x\cos x+\cos x,\;$ implying

$\displaystyle\int_{0}^{\frac{\pi}{2}}\frac{1}{\sin x+\sin x\cos x+\cos x}dx \ge\frac{1}{2}\int_{0}^{\frac{\pi}{2}}\ge\frac{\pi}{4}.$

Note that, according to wolframalpha, $\displaystyle\int_{0}^{\frac{\pi}{2}}\frac{1}{\sin x+\sin x\cos x+\cos x}dx\approx 1.02245.$